Amit Sir Please Help
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Amit Sir Please Help
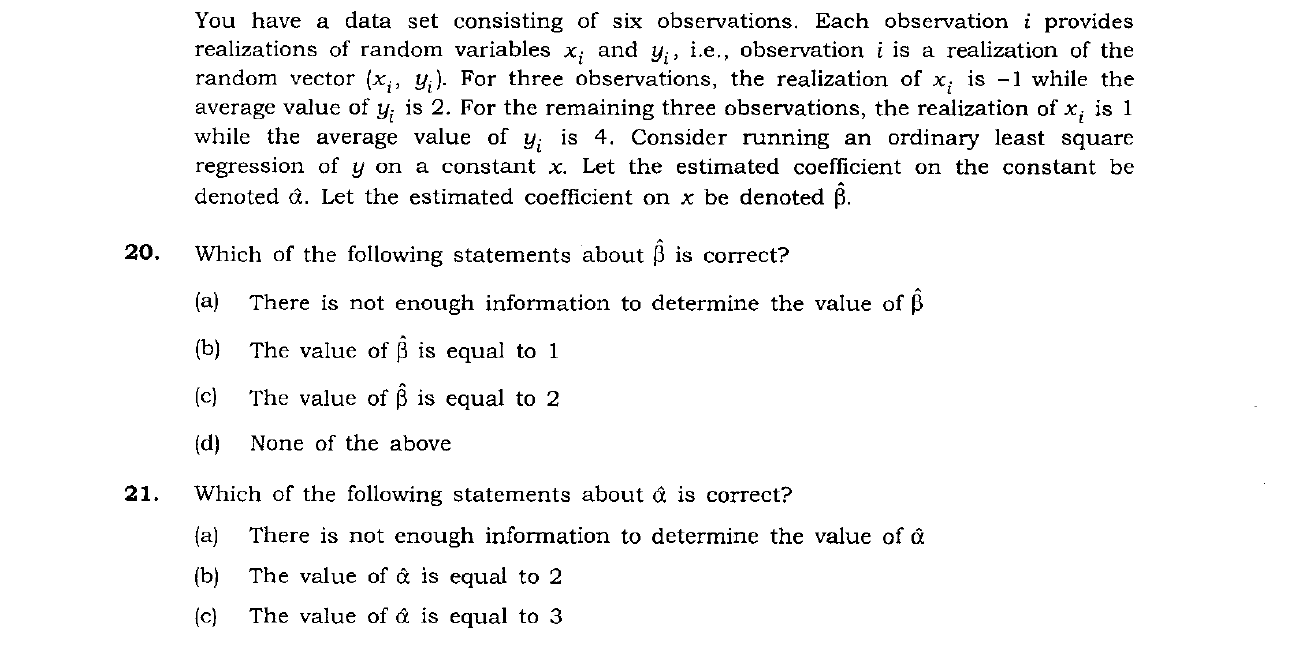  |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Amit Sir Please Help
|
Although this post is reserved for Amit sir's reply.....but I eagerly wanted to try this one.....I am getting alpha hat as 3 please confirm if it is right or wrong....
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Amit Sir Please Help
|
Vaibhav dnt knw the answer...if ur pretty sure..show the working vandita On 13 Apr 2014 14:49, "Vaibhav Garg [via Discussion forum]" <[hidden email]> wrote:
Although this post is reserved for Amit sir's reply.....but I eagerly wanted to try this one.....I am getting alpha hat as 3 please confirm if it is right or wrong.... |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Amit Sir Please Help
|
OK...I proceeded this way..... I first computed the mean of X which turns out to 0 and mean of Y which turns out to 3
Since sample regression line passes through sample means therefore Mean of y= alpha cap + beta cap*mean of x 3= alpha cap + beta cap*0 Alpha cap=3... |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Amit Sir Please Help
|
I am also getting alpha as 3.
For beta, i think answer should be not enough info is provided . because we can't find out the individual values of y from whatever is given. |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Amit Sir Please Help
|
Administrator
|
In reply to this post by vandita24x7
For Q 20 it is (b) Slope estimate is 1
For Q 21 it is (c) Intercept estimate is 3 For Q 22 it is (b) R-square is strictly above 0 |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Amit Sir Please Help
|
In reply to this post by vandita24x7
 and the slope coefficient is not 0 therefore R^2>0
Akshay Jain
Masters in Economics Delhi School of Economics 2013-15 |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Amit Sir Please Help
|
In reply to this post by vandita24x7
Which book should i refer for etx...basic etx...or essential etx???
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Amit Sir Please Help
|
Basic ecotrix....
Akshay Jain
Masters in Economics Delhi School of Economics 2013-15 |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Amit Sir Please Help
|
In reply to this post by vandita24x7
OH Thanxx ya.
I have essentials of etx...wht do i do?Do i really need to get basic etx? Eseentials wont do? Ah ..Please suggest :( |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Amit Sir Please Help
|
I have not read the essentials 1 so cant really tel but max pple say that the contents of both are more or less the same....

Akshay Jain
Masters in Economics Delhi School of Economics 2013-15 |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Amit Sir Please Help
|
Administrator
|
This post was updated on Apr 14, 2014; 1:40pm.
In reply to this post by Akshay Jain
Hi Akshay,
There is a mistake in your analysis. We are not given E(Y|X=1) and E(Y|X=-1) . These are population averages. We are only given sample averages. If the observations are (-1, y(1)), (-1, y(2)), (-1, y(3)), (1, y(4)), (1, y(5)), (1, y(6)), we only know that [y(1) + y(2) + y(3)]/3 = 2 and [y(4) + y(5) + y(6)]/3 = 4. Note that x-bar = 0. Thus, least square estimate of the slope coefficient is: b = [x(1)y(1) + x(2)y(2) + x(3)y(3) + x(4)y(4) + x(5)y(5) + x(6)y(6)]/ [x(1)^2 + x(2)^2 + x(3)^2 + x(4)^2 + x(5)^2 + x(6)^2] = [-y(1) -y(2) -y(3) + y(4) + y(5) + y(6)]/ [6] = [-6 + 12]/6 = 1 The estimate of the intercept coefficient is a = (y-bar) - b (x-bar) = y-bar = [y(1) + y(2) + y(3) + y(4) + y(5) + y(6)]/6 = [6 + 12]/6 = 3. |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Amit Sir Please Help
|
Thanks a lot sir..! vandita On 14 Apr 2014 07:57, "Amit Goyal [via Discussion forum]" <[hidden email]> wrote:
Hi Akshay, ... [show rest of quote] |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Amit Sir Please Help
|
In reply to this post by Amit Goyal
Thanku sir for pointing out.....i forgot to distinguish b/w sample reg lne and population reg line
right answers but wrong approach... 
Akshay Jain
Masters in Economics Delhi School of Economics 2013-15 |
«
Return to General Discussions
|
1 view|%1 views
| Free forum by Nabble | Edit this page |

