DSE 2011 A (a request to duck/Amit Sir, Q40 plz)
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
DSE 2011 A (a request to duck/Amit Sir, Q40 plz)
|
This post was updated on May 13, 2013; 12:50pm.
Ques no 35
 what is wrong in the following solution as the correct ans is .(b) equal to the probability that it will end in 6 games. 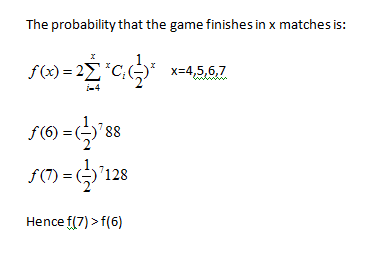
---
"You don't have to believe in God, but you should believe in The Book." -Paul Erdős |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2011 A

---
"You don't have to believe in God, but you should believe in The Book." -Paul Erdős |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2011 A

---
"You don't have to believe in God, but you should believe in The Book." -Paul Erdős |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2011 A
|
In reply to this post by Sinistral
Hi Sinistral
for the Q. 8 if u carefully calculate, u ll get solutions as = 10 (1/2)^5 for both, 6 games as well as 7 games.. :) hence option B |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2011 A
|
i think i made 1 mistake in my earlier solun.
is this solution correct??? f(6)= 2* [5C3]*(1/2)^5 * (1/2) f(7)= 2* [6C3]*(1/2)^6 * (1/2) but still f(6) not equal to f(7)
---
"You don't have to believe in God, but you should believe in The Book." -Paul Erdős |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2011 A
|
Hi sinistral...
u got it right this time... but u get the answer same for 6 and 7 matches... :) plz chek yr calculations once more ..!  Plz let me know if u figure out the rest of the two questions that u posted ! |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2011 A
|
phew!!! finally f(6)=f(7) :)
and rest of the 2 ques please...
---
"You don't have to believe in God, but you should believe in The Book." -Paul Erdős |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2011 A
|
In reply to this post by Sinistral
duck, wolverine or any one else,
could u please help me with ques no. 36 & 40
---
"You don't have to believe in God, but you should believe in The Book." -Paul Erdős |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2011 A
|
Q36. b).747
Expalanation: put 1 green ball & zero red ball in first box and 49 green balls & 50 red balls in 2nd box.. So, Prob.=1/2*(1)+1/2*49/50=0.747.
M.A Economics
Delhi School of Economics 2013-15 Email Id:sumit.sharmagi@gmail.com |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2011 A
|
In reply to this post by Sinistral
Bump. Had a problem in the same aforementioned questions!
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2011 A (a request to duck)
|
In reply to this post by Sinistral
How did you get the probability distribution function?
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2011 A
|
In reply to this post by laracroft
Could you explain how you got the answer for question 8?
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2011 A
|
Q36) Lets, suppose he puts (50 R) in Bag 1 and (50 G) in bag 2.
So, probability of A being admitted = 1/2 But, we've a better way: Put (50R + 1G) in Bag 1 and (49G) in Bag 2. Probability = (1/2)(1/51) + (1/2) But again, we've a better way: Put (50R + 2G) in Bag 1 and (48G) in Bag 2. Probability = (1/2)(2/52) + (1/2) ........ ........ and so on... Thereofore , the Best way: Put (50R + 49G) in Bag 1 and (1 G) in Bag 2 Probability = (1/2)(49/99) + (1/2) So, the idea is we can always ensure a Probability of (1/2) by keeping only the minimum number of green balls in Bag 2. And hence, can maximise the probability in the above way.
:)
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2011 A
|
thank u so much duck and Sumit
now after looking at the explanation, the distribution seems fairly logical. :) thanx once again. plz help me with student and the no. of tests he can take prob as well, Q40.
---
"You don't have to believe in God, but you should believe in The Book." -Paul Erdős |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2011 A
|
In reply to this post by Sinistral
How did you arrive at these calculations?
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2011 A
|
first of all the solution to ques no 8 given in the very first post is wrong (as it is clear by reading the subsequent replies)
the right ans is in the 4th reply to calculate how the game finishes in exactly 6 or exactly 7 matches, keep in mind that the game has to be stretched till the last match ==> the concerned player SHOULD win the LAST match AND he should have already won EXACTLY 3 of his previous matches (in this case 3 of his previous 5 or 6 matches) for example calculating for x=6 5C3(1/2)^5: choosing 3 out of his previous 5 matches and winning those (& obviously losing the remaining) 1/2: probability of winning the last match. 2 : this can be done for both the players. multiplying the above gives: f(6)= 2* [5C3]*(1/2)^5 * (1/2) similarly for f(7) note that f(6)=f(7)
---
"You don't have to believe in God, but you should believe in The Book." -Paul Erdős |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2011 A
|
Thanks mate. Cleared that up well. Cheers!
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2011 A
|
In reply to this post by Sinistral
very well explained. thumbs up
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2011 A (a request to duck/Amit Sir, Q40 plz)
|
In reply to this post by Sinistral
hey sinistral whats the answer for que 40
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2011 A (a request to duck/Amit Sir, Q40 plz)
|
ans is b
Explanation by Amit Sir A more detailed explanation
---
"You don't have to believe in God, but you should believe in The Book." -Paul Erdős |
«
Return to General Discussions
|
1 view|%1 views
| Free forum by Nabble | Edit this page |

