DSE 2013 Paper Discussion
1 ...
56789
 May 25, 2014; 7:18pm
May 25, 2014; 7:18pm
 May 25, 2014; 8:23pm
May 25, 2014; 8:23pm
 May 26, 2014; 6:34am
May 26, 2014; 6:34am
 May 26, 2014; 6:47am
May 26, 2014; 6:47am
 May 26, 2014; 10:42am
May 26, 2014; 10:42am
 May 26, 2014; 8:19pm
May 26, 2014; 8:19pm
 May 26, 2014; 9:58pm
May 26, 2014; 9:58pm
 May 27, 2014; 3:08am
May 27, 2014; 3:08am
 May 27, 2014; 7:03am
May 27, 2014; 7:03am
 May 27, 2014; 8:16am
May 27, 2014; 8:16am
 May 27, 2014; 8:32am
May 27, 2014; 8:32am
 May 27, 2014; 8:35am
May 27, 2014; 8:35am
 May 27, 2014; 8:54am
May 27, 2014; 8:54am
 May 29, 2014; 6:48pm
May 29, 2014; 6:48pm
 May 30, 2014; 5:00am
May 30, 2014; 5:00am
 May 30, 2014; 7:03am
May 30, 2014; 7:03am
 May 30, 2014; 7:58am
May 30, 2014; 7:58am
 May 30, 2014; 8:41am
May 30, 2014; 8:41am
 May 30, 2014; 2:47pm
May 30, 2014; 2:47pm
 May 31, 2014; 3:44pm
May 31, 2014; 3:44pm
1 ...
56789
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2013 Paper Discussion
|
Series 1 or Series 4..??
"I don't ride side-saddle. I'm as straight as a submarine"
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2013 Paper Discussion
|
This post was updated on May 26, 2014; 5:12am.
In reply to this post by akanksha
Q41) The consumer will be indifferent between the two bundles if the utility derived under optimum situation is equal in bothe the cases.
Now consider case 1: the optimum demand bundles are x1=x2=M/(p1+p2)=M/3. In case 2: M=px*X+py*Y, or, M=1*X+(2-θ)*Y+T. or, M-T=1*X+(2-θ)*Y. The optimum bundles in this case is given by x1=x2=(M-T)/(1+(2-θ))=(M-T)/(3-θ). Utilities will be equal implies, M/3=(M-T)/(3-θ)......................(a). from (a) we can derive, θ=3T/M (option b). Q42) The consumer will borrow in period 1 only if (1+r)<=1/β, r<=(1/β)-1, option a cannot be the answer as r will depend on the value of β, same is with option b, option c also cannot be the answer as derived, option d is the answer as it lies in the defined set r<=(1/β)-1. Q49)Use the concept that for any point which is Competitive equilibrium the monetary value of the excess demand will be zero..this leads to the condition that the market gets cleared, so competitive a competitive price ratio will clear the market...now the question remains how to find that price ratio..under the assumption that the preferences are monotone, complete and convex a Pareto efficient bundle leads to a competitive equilibrium (second theorem of welfare economics)..now we know that a Lexicographic preference ordering is monotone, complete and convex so a choose a Pareto Efficient point and compare..in this case the initial endowment bundle is Pareto efficient (you can check that too). This gives. px*10+py*0=px*0+py*10. or, px=py. or, px/py=1. So option b seems to be the only answer because option a may or may not be true because px=py is true but it may or may not be equal to 1. Option c and d cannot be true because px=py, so both px>py and py>px are false..so option c suits best.
"I don't ride side-saddle. I'm as straight as a submarine"
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2013 Paper Discussion
|
Subhayu..pls guide me fr where to do intertemporal ?
MA Economics
DSE 2014-16 |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2013 Paper Discussion
|
I think that for any of the Micro topics Varian is the best book from where you can get the concepts right..starting from Utility to Welfare (General Equilibrium)...going thoroughly between the lines and understanding the concepts is the best way which has been dealt in best manner in Hal. R. Varian..I think that will help you rather than going for any higher level text.
"I don't ride side-saddle. I'm as straight as a submarine"
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2013 Paper Discussion
|
thank you

MA Economics
DSE 2014-16 |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2013 Paper Discussion
|
In reply to this post by Akshay Jain
hi Akshay for q 35. i am getting option (a) i.e always pareto efficient but the correct answer is (d) plz explain... thanx
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2013 Paper Discussion
|
In reply to this post by kangkan
@kangkan for q 38->
. (Suppose that a city can be described by an interval [0, 1]. Only two citizens, A and B, live in this city at different locations; A at 0.2 and B at 0.7. Government has decided to set up a nuclear power plant in this city but is yet to choose its location. Each citizen wants the plant as far as possible from her home and hence both of them have the same utility function, u(d) = d, where d denotes the distance between the plant and home. Find the set of Pareto optimal locations for the plant. (a) All locations in the interval [0, 1] are Pareto optimal (b) All locations in the interval [0.2, 0.7] are Pareto optimal (c) 0.5 is the only Pareto optimal location (d) 0 and 1 are the only Pareto optimal locations) b must be the correct any idea....??? 
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2013 Paper Discussion
|
suppose that A's utility function is U(d)a=da and utility function of B is U(d)b=db
where da = |plant location in [0,1] - 0.2| and db = |plant location in [0,1] - 0.7| If the plant is at extreme position say 0 den da= 0.2, db=0.7 and when plant is at extreme position 1 dn da=0.8, db=0.3 now take a plant location in interval (0,0.2) dn da<0.2 and db<0.7, so both of them are worse off (position 0 pareto dominates (0,0.2) similarly take a plant location in interval (0.7,1) dn da<0.8 and db<0.3, so both of them are worse off (position 1 pareto dominates (0.7,1) therefore option (a) is ruled out now take a location 0.5 dn da=0.3 and db=0.2 which means utility of A increases but dat of B decreases when compared with location 0 but it is pareto dominated by location 1 so option b and c are also ruled out hence option D
Akshay Jain
Masters in Economics Delhi School of Economics 2013-15 |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2013 Paper Discussion
|
@Akshay..the question on heteroscekadascity..question 49 of series 1....
Accd to the big formula in gujrati(Appendix of chapter 11 page 434),the estimated vale of Sigma Hat is an unbiased estimator of sigma regardless of its relation to X(i)...but accd to answer key of of Amit Sit,it is option C...am i interpretting the the result wrongly? |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2013 Paper Discussion
|
Yes it is option c
but m not getting what is your doubt exactly???
Akshay Jain
Masters in Economics Delhi School of Economics 2013-15 |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2013 Paper Discussion
|
Hi...gujrati page 396 says that var B hat is a biased estimator of Beta in case of heteroskedascity...

|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2013 Paper Discussion
|
var (B hat)= sigma (xi^2)(si^2)/(sigma xi^2)^2 where s is sigma for variance
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2013 Paper Discussion
|
And alrenately,if Z is variable that sytematically effect the variances of ui and has been left out,isnt the model underfitted?and if its undefitted,accd to what i cud make out from gujati(whcih isnt a lot :( ) ,is that that variance of sigma hat will be a biased estimator of actual sigma
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2013 Paper Discussion
|
Please help me with Q 42, 45, 54 and 55. (Series 1)
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2013 Paper Discussion
|
Q.54, C=0.25x, D=0.75x, and R/D=0.20, also C/D=0.25/0.75, now money multiplier=(C/D+1)/(C/D+R/D)=[(0.25/0.75)+1)/(0.25/0.75)+0.20)]=0.25...so increase in money supply=2000*2.5=5000 units.
Q.55 take LM curve vertical in exogenous case and upward sloping in endogenous case. You will find (c) as your answer. Rest even I don't know... Plz reply if u know any of the answer.. http://discussion-forum.2150183.n2.nabble.com/DSE-2013-Doubts-td7590991.html#a7591065 |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2013 Paper Discussion
|
In reply to this post by neha:)
Hi Neha,
Q 42: Just find the total expected value. P(Two 1 dollers)=6/20 P(One 1 doller, One 4 doller)=6/10 P(Two 4 dollers)=2/20 Expected value is (6/20)*2 + (6/10)*5 + (2/20)*8=4.4 Q 44: Total ways of forming the group without restriction= 5C2 * 5C3=100 The number of ways of forming a team with Mrs.F and Mr.M in the team is 4C1 * 4C2=24 (assume M and F are alredy in the team and u have to choose the remaining team members). Total ways of forming the group without F and M in the team=100-24=76 |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2013 Paper Discussion
|
@ Viv and Phelps : Thanks a lot!
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2013 Paper Discussion
|
How to do 58th? series 1..

|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2013 Paper Discussion
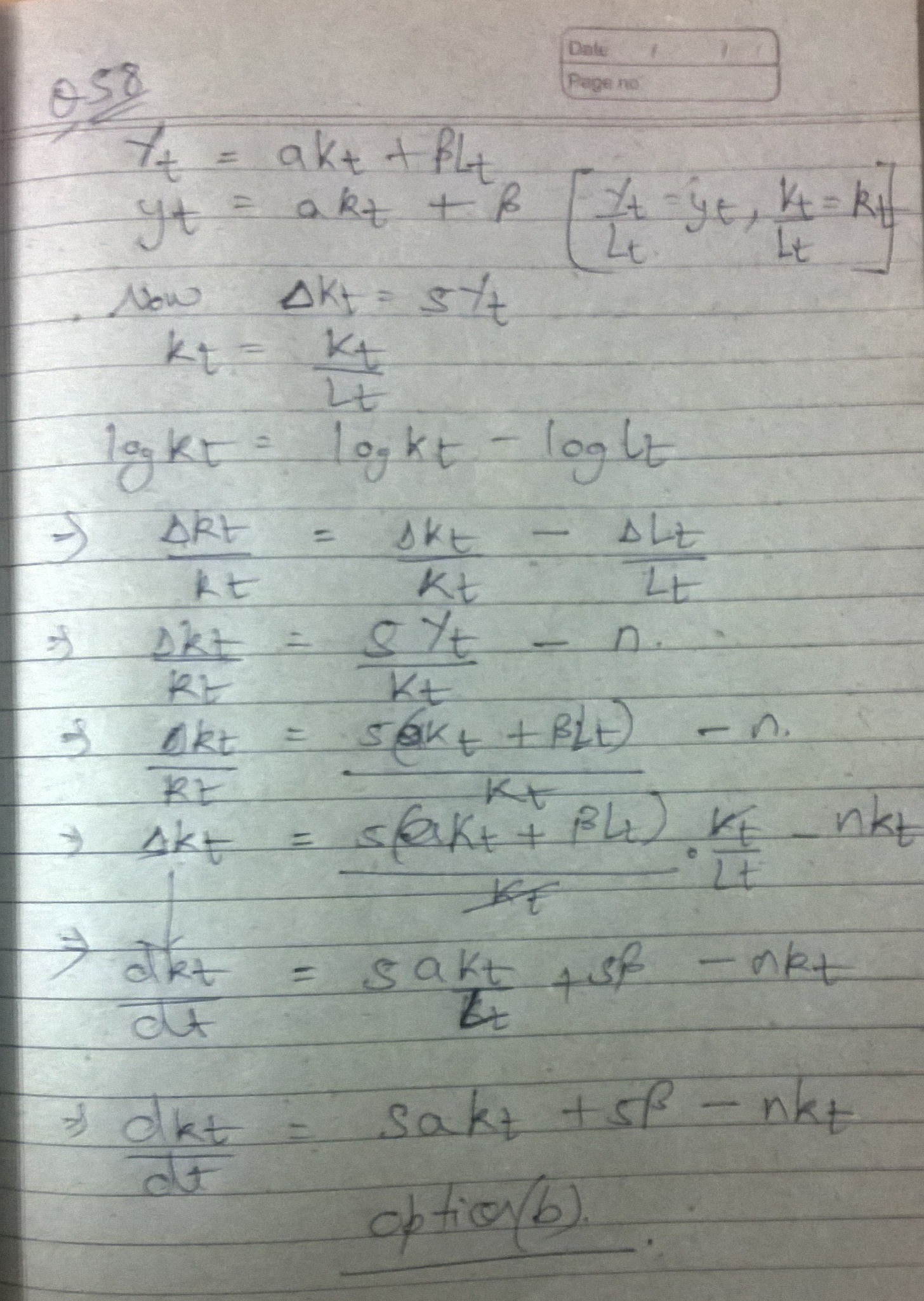
Akshay Jain
Masters in Economics Delhi School of Economics 2013-15 |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2013 Paper Discussion
|
Thanks a lot! Really helpful!
Can you explain the next step after the log equation? |
«
Return to General Discussions
|
1 view|%1 views
| Free forum by Nabble | Edit this page |

