Demand question

[lucy]

[onionknight]

Indifference curves for the given utility function look like this:


The given consumption bundle gives the consumer a utility of 32 and lies on the part of the indifference curve with slope 2. If the consumer maximizes utility, he would choose a consumption bundle on the line with slope 2 only if the price ratio is also 2. Py=0.5 and price ratio=2 implies Px=1. Income = 1*8+0.5*16=16

[lucy]

thanks.
Any idea about this one?

[bhavna khari]

Hii
Which book is this ?

On May 23, 2015 10:54 PM, "lucy [via Discussion forum]" <[hidden email]> wrote:

thanks.
Any idea about this one?

---

If you reply to this email, your message will be added to the discussion below:

http://discussion-forum.2150183.n2.nabble.com/Demand-question-tp7597108p7597162.html

To start a new topic under General Discussions, email [hidden email]
To unsubscribe from General Discussions, click here.
NAML

[onionknight]

The budget line is just c+s=I where I is the income. Slope of the indifference curve is 10000/c. Indifference curves look like this:

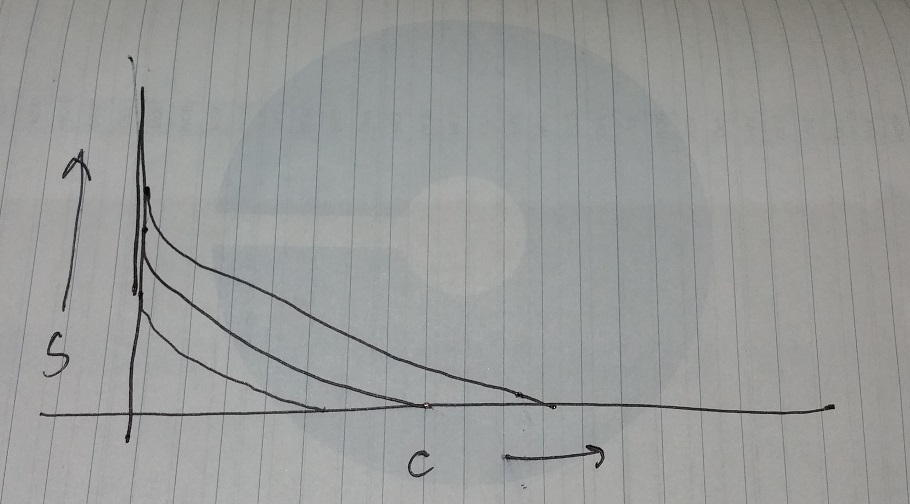
Slope where the indifference curve touches the y axis is infinity and where it touches the x axis, the slope takes some finite value(10,000/c). Slope of the budget line is 1. The optimal bundle is located on the x axis until c=e and after that it is located somewhere in the first quadrant such that 10000/c=1 or c=10,000 and the rest is saved.Thus, option A is correct.