Discussion Problem_(21)
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Discussion Problem_(21)
|
Suppose, joint probability density fucntion (pdf) of is given by:
f(x,y) = 2 for 0<x<y<1. = 0 otherwise. Find P(X+Y≤1).
:)
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Discussion Problem_(21)
|
let u=x+y; w=y
==> x=u-w & y=w |J|=1 f(u,w)=2|J|=2 , 0<w<u<2w<2 = 0 , e.w. f_u(u)= integral (from u/2 to u) f(u,w) dw (as w lies between u/2 and u) where f_u(u) is the marginal pdf of u =2u/2 =u 0<u<2 P(X+Y≤1)= P(U<=1)= integral (from 0 to 1) f_u(u) du = integral (from 0 to 1) u du = 1/2
---
"You don't have to believe in God, but you should believe in The Book." -Paul Erdős |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Discussion Problem_(21)
|
In reply to this post by duck
I just thought it can be done in a simpler way:
P(X+Y<=1) = Integral (from x=0 to 1/2) Integral (from y=x to 1-x) f(x,y) dy dx Since: (double integration limits can be seen by superimposing x<y graph on x+y<=1 graph and hashing the required area) = 1/2
---
"You don't have to believe in God, but you should believe in The Book." -Paul Erdős |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Discussion Problem_(21)
|
In reply to this post by duck
what will the limits of the integral be if we fix y instead of y.?
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Discussion Problem_(21)
|
i am sorry .
i mean the limits when we fix y instead of x .? |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Discussion Problem_(21)
|
0 to 1-y for 0.5<y<1 and 0 to y for 0<y<0.5. you'll have a sum of two integrals in this case.
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Discussion Problem_(21)
|
In reply to this post by Mauli
Great!
Correct Answer = 1/2 @Mauli: If we fix y then, there will be two triangle over which you'll integrate. Limits are clearly mentioned by Vasudha in this case.. :)
:)
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Discussion Problem_(21)
|
Im not getting the ans! x to 1-x, what value do we out in the integral?
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Discussion Problem_(21)
|
In reply to this post by Sinistral
hey sinistral.... i did not understand this one.... kindly explain.... how exactly did u arrive at the solution !!???
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Discussion Problem_(21)
|
find the area of the shaded triangle (figure below) and multiply it by f(x,y) ie 2. u will get 1/2
or u can integrate as done here Integration is done over shaded area as only in this region x<y and x+y<1 it can be seen from the graph that as x goes from 0 to 1/2 => y goes from blue line to red line. blue line is y=x and red line is y=1-x. so limit of y will go from x to 1-x 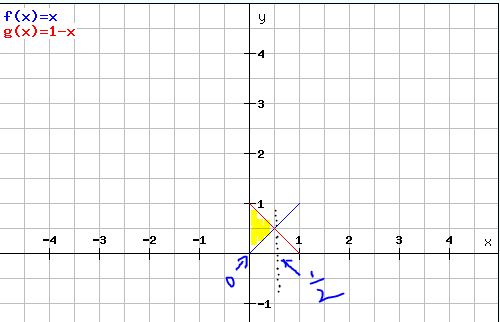
---
"You don't have to believe in God, but you should believe in The Book." -Paul Erdős |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Discussion Problem_(21)
|
In reply to this post by Sinistral
i know this will seem silly, but plz tell me how did u arrive at this limit of 1/2 for x ??
 |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Discussion Problem_(21)
|
beyond x=1/2 we will go outside the required triangle. So x=1/2.
x=1/2 is the point where blue line (y=x) and red line (y=1-x) intersect. equating the above 2: x=1-x ==> x=1/2
---
"You don't have to believe in God, but you should believe in The Book." -Paul Erdős |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Discussion Problem_(21)
|
oh yeah.... thanku sooo much !
i am so sorry to have bothered u with such a silly question... plz excuse me..  bt thanx, nevertheless! |
«
Return to General Discussions
|
1 view|%1 views
| Free forum by Nabble | Edit this page |

