'Examine if estimator is unbiased'
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
'Examine if estimator is unbiased'
|
A random variable X has a continuous uniform distribution over the interval from 0 to θ, where θ is an unknown parameter. If the Sum of the largest and smallest values of X in the sample of n observations of X is used to estimate θ, explain whether or not the estimator is (1) unbiased (2) consistent.
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: 'Examine if estimator is unbiased'
|
it is unbiased..do not know about inconsistecy...check out the section on order statistics of freud(chapter 8)
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: 'Examine if estimator is unbiased'
|
sorrry...i8ts biased
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: 'Examine if estimator is unbiased'
|
This post was updated on May 29, 2014; 4:01am.
In reply to this post by Nupur
CONTENTS DELETED
The author has deleted this message.
"I don't ride side-saddle. I'm as straight as a submarine"
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: 'Examine if estimator is unbiased'
|
Why is E(theta square) = theta square?
“Operator! Give me the number for 911!”
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: 'Examine if estimator is unbiased'
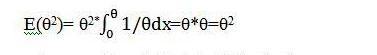
"I don't ride side-saddle. I'm as straight as a submarine"
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: 'Examine if estimator is unbiased'
|
In reply to this post by Granpa Simpson
Subhayu why have you fixed x-min and x-max as 0 and theta? The minimum and maximum value will differ from sample to sample and as such they should be random variables?? Take note of the fact that the sum of smallest and largest values is a sample statistic..
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: 'Examine if estimator is unbiased'
|
Yeah, thats my point too. The max and the min values will vary sample to sample.
So the sample statistic becomes Theta hat (call it $) = Now E($) = E( min (x1, x2, xn) ) + E( Max (x1,x2..... xn) ) = something => 0 + ( Something <= theta) which is not exactly equal to theta. But it will tend to theta as n tends to infinity. Therefore $ is consistent but not unbiased. I dont know I am right or wrong. |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: 'Examine if estimator is unbiased'
|
In reply to this post by Nupur
yes ur right..i didnt note that...in that case my method is wrong..!!!
"I don't ride side-saddle. I'm as straight as a submarine"
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: 'Examine if estimator is unbiased'
|
In reply to this post by ashutosh
But the answer is Unbiased and Consistent.
Here is the explanation that is given for unbiasedness: "The estimator will be unbiased. Call the maximum value of X in the sample Xmax and the minimum value Xmin. Given the symmetry of the distribution of X, the distributions of Xmax and Xmin will be identical, except that that of Xmax will be to the right of 0 and that of Xmax will be to the left of θ. Hence, for any n, E(Xmin)-0=θ-E(Xmax) and the expected value of their sum is equal to θ." Now what I don't understand here is the equation in bold above!! Can someone please explain this? |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: 'Examine if estimator is unbiased'
|
Okay! Right.
That thing in bold is little confusing but seems right. I made an error in my answer. It should be like this- Statistic is $ = min (x1, x2, xn) ) + Max (x1,x2..... xn) Now E($) = E( min (x1, x2, xn) ) + E( Max (x1,x2..... xn) ) = something => 0 + ( Something <= theta) = ( 0+ e) + ( theta - e) (where e is epsilon. E ($) = theta. hence unbiased and consistent. |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: 'Examine if estimator is unbiased'
|
Thank you Ashutosh and Subhayu!
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: 'Examine if estimator is unbiased'
|
Ur welcome Nupur..!!!nywa my solution was wrong....
 ....thanx to u for posting a good problem..and thanx to Asutosh for providing the solution... ....thanx to u for posting a good problem..and thanx to Asutosh for providing the solution... 
"I don't ride side-saddle. I'm as straight as a submarine"
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: 'Examine if estimator is unbiased'
|
Administrator
|
In reply to this post by Nupur
Detailed Solution is attached:
sol.png |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: 'Examine if estimator is unbiased'
|
Sir, thanks for the detailed and remarkable solution!
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: 'Examine if estimator is unbiased'
|
Administrator
|
Most welcome :)
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: 'Examine if estimator is unbiased'
|
Thanks a lot sir :)
Just a doubt sir. Is it important to check for consistency once we know that an estimator is unbiased. Can there be cases in which some estimator is unbiased but not consistent ? |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: 'Examine if estimator is unbiased'
|
Administrator
|
Unbiasedness and consistency are two very different properties. Unbiasedness property says that the estimator on an average delivers the true value. Consistency says that as sample size increases the probability that the estimators delivers the value very different from the true one goes to zero. In other words, it delivers the value very close to true value with almost certainty when the sample size is high.
For example, in the problem that is considered above where we were trying to estimate theta: Y = max{X(1), ... , X(n)} is a biased but consistent estimator of theta. And T = X(1) + X(n) is an unbiased but not a consistent estimator of theta. |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: 'Examine if estimator is unbiased'
|
thankyou sir :)
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: 'Examine if estimator is unbiased'
|
In reply to this post by Amit Goyal
Thanx a lot sir..:)
"I don't ride side-saddle. I'm as straight as a submarine"
|
«
Return to General Discussions
|
1 view|%1 views
| Free forum by Nabble | Edit this page |

