ISI 2007 ME-I - Doubts
12
 May 07, 2014; 1:42pm
May 07, 2014; 1:42pm
 May 07, 2014; 2:55pm
May 07, 2014; 2:55pm
 May 07, 2014; 3:04pm
May 07, 2014; 3:04pm
 May 07, 2014; 6:09pm
May 07, 2014; 6:09pm
 May 07, 2014; 6:15pm
May 07, 2014; 6:15pm
 May 07, 2014; 6:22pm
May 07, 2014; 6:22pm
 May 07, 2014; 7:24pm
May 07, 2014; 7:24pm
 May 08, 2014; 7:59pm
May 08, 2014; 7:59pm
 May 08, 2014; 8:02pm
May 08, 2014; 8:02pm
 May 09, 2014; 8:37am
May 09, 2014; 8:37am
 May 09, 2014; 8:46am
May 09, 2014; 8:46am
 May 09, 2014; 8:50am
May 09, 2014; 8:50am
 May 09, 2014; 9:09am
May 09, 2014; 9:09am
 May 09, 2014; 9:43am
May 09, 2014; 9:43am
 May 09, 2014; 9:56am
May 09, 2014; 9:56am
 May 09, 2014; 10:04am
May 09, 2014; 10:04am
 May 09, 2014; 10:10am
May 09, 2014; 10:10am
 May 09, 2014; 10:21am
May 09, 2014; 10:21am
 May 09, 2014; 11:14am
May 09, 2014; 11:14am
 May 09, 2014; 11:29am
May 09, 2014; 11:29am
12
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
ISI 2007 ME-I - Doubts
|
Question paper - http://economicsentrance.weebly.com/uploads/1/1/0/5/1105777/isi2007.pdf
Q8) if P= log(base x)xy and Q = log(base y)xy then P+Q = A)PQ B) P/Q C) Q/P D) (PQ)/2 Q12) - I got (A) exactly but Amit sirs solution says B -- someone please explain how? http://economicsentrance.weebly.com/uploads/1/1/0/5/1105777/isi2007.pdf Q14) What does notation f(x;1) and f(x;0) mean? Q21) Again I got (A) = 1 .. please explain how the answer is B? 23) How to approach this question?! 25) Again I got 240 possibilities.. please explain how the answer is 168 27) M=n/2 I get that.. but how to calculate variance? 28) ?? They all seem correct to me!! What am I missing? Please respond!! Thank you! |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: ISI 2007 ME-I - Doubts
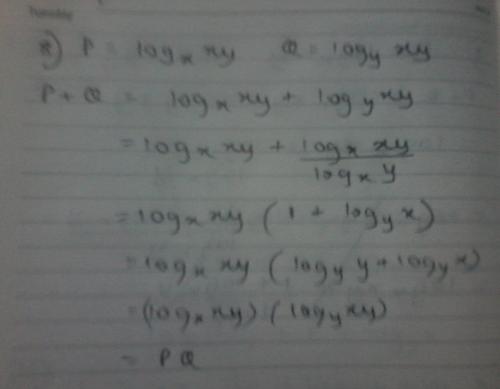 For 12, take log on both sides and then differentiate wrt x. put x = 0 , you will get (B)
“Operator! Give me the number for 911!”
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: ISI 2007 ME-I - Doubts
|
Thanks a lot Tsuki..
For 12 I differentiated directly and got (A).. why is it wrong to differentiate directly? |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: ISI 2007 ME-I - Doubts
|
In reply to this post by Ridhika
Q14) Here, f(x;θ)=θ*f(x;1)+(1-θ)*f(x;0), where both f(x;1) and f(x;0) are pdf’s,
Now, ∫ f(x;θ)dx= θ*∫ f(x;1)dx+ (1-θ)*∫ f(x;0)dx. Since ∫ f(x;1)dx=∫ f(x;0)dx=1. ∫ f(x;θ)dx=θ+(1-θ)=1. Hence option d, f(x;θ) is a pdf for every θ Q21) Integrate the function over (0,1), then (1,sqrt(2)), then (sqrt(2),3/2)) and you will get b as answer.
"I don't ride side-saddle. I'm as straight as a submarine"
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: ISI 2007 ME-I - Doubts
|
In reply to this post by Ridhika
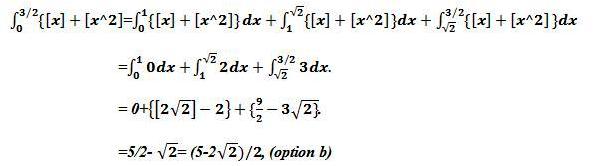
"I don't ride side-saddle. I'm as straight as a submarine"
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: ISI 2007 ME-I - Doubts
|
In reply to this post by Ridhika
Q23) Just use normal algebraic equation and then compare the options, dats the easiest way to get the answer, u will get a relationship in the form of this identity (p1+2*p2) = 750*p1*p2, compare the options and u will get that both p1=p2 and p2=(3/4)p1 satisfies the identity.
"I don't ride side-saddle. I'm as straight as a submarine"
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: ISI 2007 ME-I - Doubts
|
In reply to this post by Ridhika
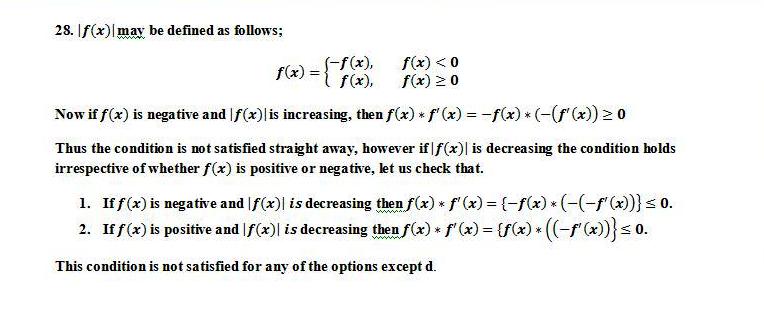
"I don't ride side-saddle. I'm as straight as a submarine"
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: ISI 2007 ME-I - Doubts
|
Thanks a lot Subhayu.. got these!!
 Also I think you have posted the ans to 29 instead of 28.. |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: ISI 2007 ME-I - Doubts
|
for Q28..all of the options seems to go with the problem given..

"I don't ride side-saddle. I'm as straight as a submarine"
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: ISI 2007 ME-I - Doubts
|
Question 21, could someone share the workings for integral of fractional function.
Thanks |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: ISI 2007 ME-I - Doubts
|
The working has been shared..u just check the previous posts..!!
"I don't ride side-saddle. I'm as straight as a submarine"
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: ISI 2007 ME-I - Doubts
|
In reply to this post by rongmon
Just noticed above workings.
thanks. |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: ISI 2007 ME-I - Doubts
|
In reply to this post by Granpa Simpson
Subhayu, could you briefly elaborate on how you derived the second step in the solution
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: ISI 2007 ME-I - Doubts
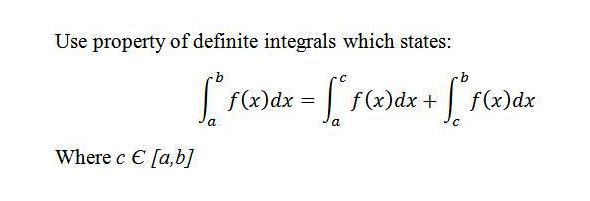
"I don't ride side-saddle. I'm as straight as a submarine"
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: ISI 2007 ME-I - Doubts
|
Right, how does that lead to the next equation exactly?
Sorry for the bother! |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: ISI 2007 ME-I - Doubts
|
In the interval [0,1], [x] =[x^2]=0, in the interval [1, sqrt(2)], [x]=[x^2]=1 and in the interval [sqrt(2), 3/2], [x]=1 and [x^2]=2.
"I don't ride side-saddle. I'm as straight as a submarine"
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: ISI 2007 ME-I - Doubts
|
In reply to this post by rongmon
Its not at all bothering brother..absolutely ok..!
"I don't ride side-saddle. I'm as straight as a submarine"
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: ISI 2007 ME-I - Doubts
|
Grateful!
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: ISI 2007 ME-I - Doubts
|
I got 25
 number 3 can only be in 3rd , 4th or 5th positions as it must be preceeded and suceeded by 2 numbers. Now if it is in 3rd place -> it can be preceeded in 2! ways and succeded in 4! ways. So total = 48. Exactly the reverse argument holds when 3 is in 5th place. So again there are 48 cases. When 3 is in 4th place .. there are 3 places before and 3 after. in the 3 places before 1 and 2 must be there.. so we need to do 3P2 = 6. similarly, in the 3 places after 3 we can do 3P2 for finding correct places of 6 and 7.. so we have 6*6=36 ways so far and in addition the 2 empty places can be filled by 4 or 5.. so 36*2=72 now 48 + 48 + 72=168 I hope this helps someone :D |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: ISI 2007 ME-I - Doubts
|
In reply to this post by Granpa Simpson
Hi Subhayu, can you please explain this question again?
|
«
Return to General Discussions
|
1 view|%1 views
| Free forum by Nabble | Edit this page |

