ISI 2014 PEA Answer Key
123
 May 05, 2014; 9:25pm
May 05, 2014; 9:25pm
 May 06, 2014; 10:18am
May 06, 2014; 10:18am
 May 06, 2014; 12:00pm
May 06, 2014; 12:00pm
 May 06, 2014; 6:50pm
May 06, 2014; 6:50pm
 May 06, 2014; 7:14pm
May 06, 2014; 7:14pm
 May 08, 2014; 4:41pm
May 08, 2014; 4:41pm
 May 08, 2014; 6:02pm
May 08, 2014; 6:02pm
 May 08, 2014; 11:45pm
May 08, 2014; 11:45pm
 May 09, 2014; 4:20am
May 09, 2014; 4:20am
 May 09, 2014; 4:59am
May 09, 2014; 4:59am
 May 09, 2014; 5:30am
May 09, 2014; 5:30am
 May 09, 2014; 10:42am
May 09, 2014; 10:42am
 May 09, 2014; 1:44pm
May 09, 2014; 1:44pm
 May 09, 2014; 1:49pm
May 09, 2014; 1:49pm
 May 09, 2014; 2:00pm
May 09, 2014; 2:00pm
 May 09, 2014; 2:04pm
May 09, 2014; 2:04pm
 May 09, 2014; 8:53pm
May 09, 2014; 8:53pm
 May 10, 2014; 3:30am
May 10, 2014; 3:30am
 May 10, 2014; 4:29am
May 10, 2014; 4:29am
 Feb 17, 2015; 6:51am
Feb 17, 2015; 6:51am
123
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: ISI 2014 PEA Answer Key
|
Pr{Min(X,Y)<=Z} = Pr(X<=Z,Y<=Z)=Pr(X<=Z)*Pr(Y<=Z) since the are independent,
so Pr{Min(X,Y)<=Z}=Z*Z=Z^2, Proceeding in similar manner I am getting Pr{Max(X,Y)<=(1-Z)}=(1-Z)^2, Now (1-Z)^2=Z^2, using this I am getting Z=1/2 as the answer...Amit sir plz let me know where am I am doing the mistake..!!!!
"I don't ride side-saddle. I'm as straight as a submarine"
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: ISI 2014 PEA Answer Key
|
In reply to this post by Arushi
For q 25..it is one of d standard prob based on "'Pigeon hole principal' ."
soluiton in detail :max n min no of handshakes(HSs) a person can do = 19 & 1 respectively (u can't handshake urself.) Assume for a moment, a random person ,say , A shook hand wid B n leaves d party implying A being one of d person to have shaken hand in odd number i e 1 in dis case. now, max HS B can do is 19(18 +1) while for odrs would be 18 (since A is gone home,nly 19 members r left) For d sake of argument,to make all HS even, lets say dat B does HS wid wid 17 ppl in party leaving C, dat way his total handshakes are 17 + 1 = 18 now. Now out of18 ppl( excluding A B ) , odr than C, everyone HS wid 17 making der total HS to 18 .(still even). but for B, d max HS can only b 17 ( A is gone n B refused) making his HS always odd.in total A n C have shaken hand wid odd ppl. Dis way, der cant be '1' person to have shaken hand wid odd no off ppl,wtever way u try. Since 1 cant b answer, option D is not d answer implyiing option C as answer.( u dun need to check for 19, sicne der is only 1 correct ans)... For more details, u can refer to 'Pigeon hole principal' . |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: ISI 2014 PEA Answer Key
|
Questions 6, 8 and 12 anyone?
Thank you. |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: ISI 2014 PEA Answer Key
|
This post was updated on May 06, 2014; 7:21pm.
Q6) The matrix is called p-special if detA is not divisible by p..DetA=-(a*b)....now any number can be broken down in to product of prime numbers. This number will be divisible by p only if p is one of the factors of -(a*b) or (a*b)=0. So in order to form a p-special matrix we will have to select a and b in such a way that none of them has p as a factor or their product not equal to 0. Now if you look at the set then it will be clear that since both a and b are less than p except 0 you can take any of the (p-1) elements for a and similarly (p-1) elements for b.(taking 0 there are total of p elements). Thus (a*b) can be taken in (p-1)*(p-1)=(p-1)^2 ways.
Q8) If you look at the equation then both are of odd degree, their sum can be zero only if they are equal and opposite in sign. This can occur only if x lies in [a,b] s.t it is equidistant from both a and b, thus x=(a+b)/2 is the only solution that you can get. Thats the way I have solved, however you may use theorems related to polynomials.Alternative Method: Differentiate the function wrt you will get, (2n+1)*[(x-a)^(2n)+(x-b)^(2n)], now since (2n+1) not equal to 0 and 2n is even for all n so f'(x) cannot be zero for any x, so there is no real root for f'(x) so f(x) can have either 1 or no real root, however since the polynomial is of odd degree it has atleast one real root, so it has exactly one root.
"I don't ride side-saddle. I'm as straight as a submarine"
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: ISI 2014 PEA Answer Key
|
In reply to this post by rongmon
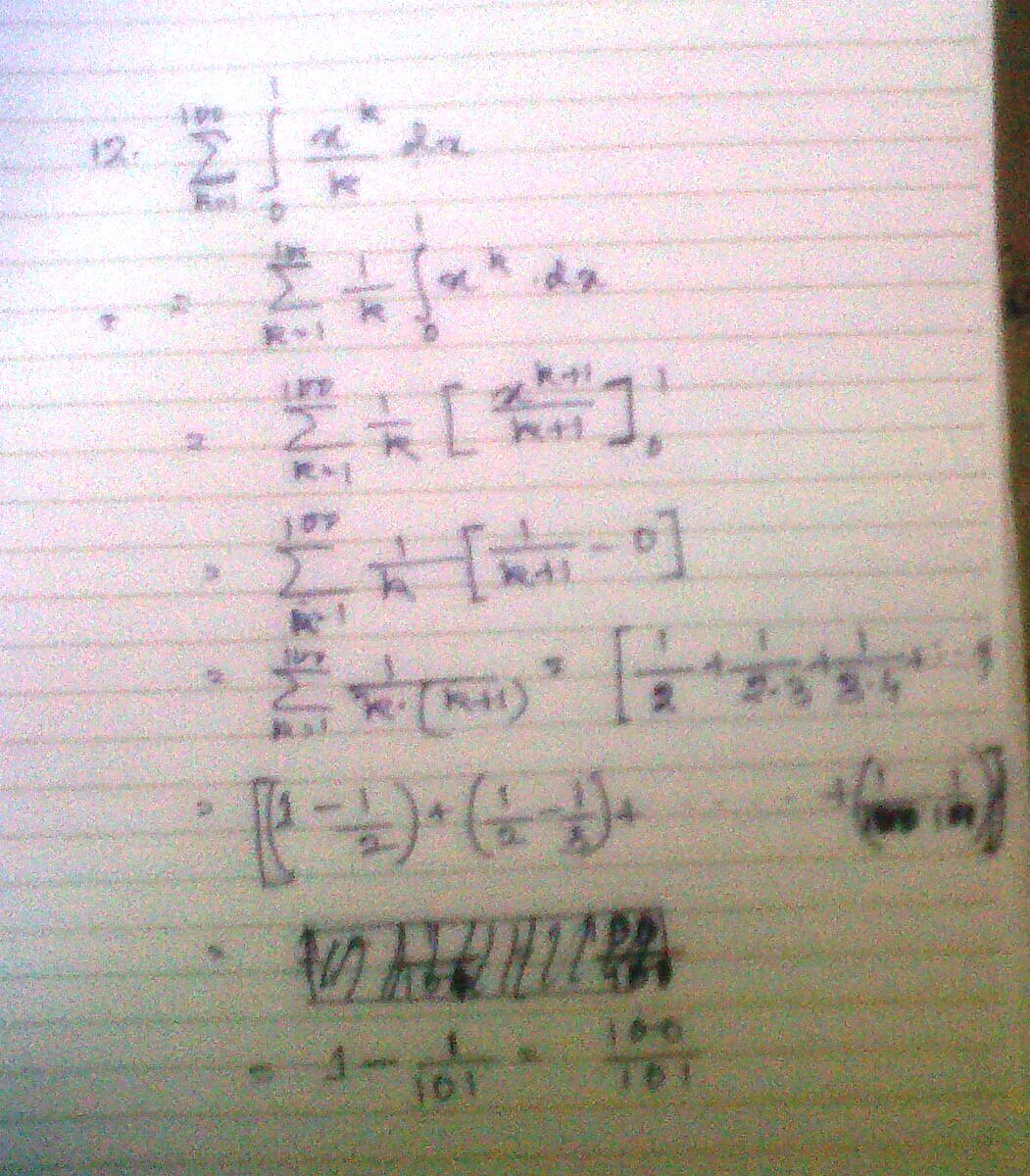
"I don't ride side-saddle. I'm as straight as a submarine"
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: ISI 2014 PEA Answer Key
|
In reply to this post by Amit Goyal
can someone help me with q 23
thanks |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: ISI 2014 PEA Answer Key
|
In reply to this post by Granpa Simpson
pls explain ques 3
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: ISI 2014 PEA Answer Key
|
In reply to this post by Granpa Simpson
Please can anyone help me with Q13? Also if you can mention from where to read for such questions.
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: ISI 2014 PEA Answer Key
|
In reply to this post by neha 1
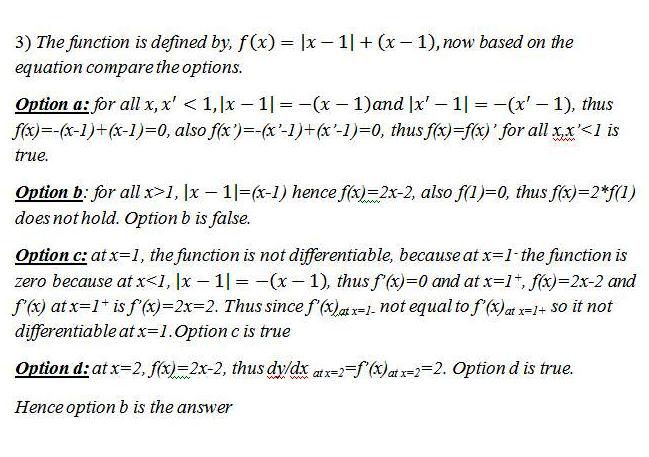
"I don't ride side-saddle. I'm as straight as a submarine"
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: ISI 2014 PEA Answer Key
|
In reply to this post by Spiti
Q13) Arrange the equation in matrix form of the form AX=b, form the augmented matrix by incorporating the constant terms. Now compare the coefficient matrix and the augmented matrix, by comparing you will find out that the Rank of Coefficient Matrix =4 and the Rank of Augmented Matrix=5 whatever be the value of alpha. The necessary and sufficient condition for a solution to exist is Rank of Coefficient Matrix=Rank of Augmented Matrix. Here this condition does not hold for any value of alpha...hence option d is the answer.
"I don't ride side-saddle. I'm as straight as a submarine"
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: ISI 2014 PEA Answer Key
|
In reply to this post by shietal
Q23) The equation to have two unique roots there can be two cases
Case 1: the equation is of the form f(x)=(x-1)*(x-a)^2, for this to hold alpha = (49/4), which is not given in the options. Case 2: f(x)=(x-1)*(x-1)*(x-a), in this case one of the roots is 1 which occurs twice and the other is a, for this to hold alpha=6. thus answer is option a.
"I don't ride side-saddle. I'm as straight as a submarine"
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: ISI 2014 PEA Answer Key
|
In reply to this post by Granpa Simpson
Hey Subhayu.. So this condition [condition for a solution to exist is Rank of Coefficient Matrix=Rank of Augmented Matrix] I know hold for a system of linear equations.. But does it always hold for a system of inequalities as well?
Also Im not being able to solve 11, 20 and 27.. Please help? |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: ISI 2014 PEA Answer Key
|
Vaibhav has posted the right solution to Q13 is this link...https://www.facebook.com/groups/659129117445776/, thanx Vaibhav..check it..!!!!
"I don't ride side-saddle. I'm as straight as a submarine"
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: ISI 2014 PEA Answer Key
|
My pleasure bro....:-)
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: ISI 2014 PEA Answer Key
|
In reply to this post by Ridhika
Ridhika....in q11...fx is PDF...therefore total area under fx should be sum to 1
So when u integratee it...ie x from 0 to a and 1/2 from a to 2 their sum should be equal to 1 You'll get a=0,1 now a cannot be 0 as prob with x<=a is positive and rv is b/w 0 and 2 only..therefore a is 1 Now Prob(X>=1) = integration ½ from 1 to 2... |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: ISI 2014 PEA Answer Key
|
@Vaibhav, please help here
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: ISI 2014 PEA Answer Key
|
In reply to this post by Granpa Simpson
Can you please post the solution in this forum, for the benefit of those who are not in the facebook group
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: ISI 2014 PEA Answer Key
|
In reply to this post by Dreyfus
Thanks a lot vaibhav ! :) and good luck for the exam tomorrow!
----- Reply message -----
From: "Vaibhav Garg [via Discussion forum]" <ml-node+[hidden email]> To: "Ridhika" <[hidden email]> Subject: ISI 2014 PEA Answer Key Date: Fri, May 9, 2014 7:30 PM Ridhika....in q11...fx is PDF...therefore total area under fx should be sum to 1
So when u integratee it...ie x from 0 to a and 1/2 from a to 2 their sum should be equal to 1 You'll get a=0,1 now a cannot be 0 as prob with x<=a is positive and rv is b/w 0 and 2 only..therefore a is 1 Now Prob(X>=1) = integration ½ from 1 to 2... If you reply to this email, your message will be added to the discussion below:
http://discussion-forum.2150183.n2.nabble.com/ISI-2014-PEA-Answer-Key-tp7587867p7589167.html
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: ISI 2014 PEA Answer Key
|
In reply to this post by Spiti
Spiti...for q13...add equation 2 and equation 4 u'll get x4-x3<=a-2
And we are given 5th eq as x4-x3<=-4 Since, this system of equation has solution that means these two inequalities needs to be consistent na for this a-2=-4 a=-2 thus option d nonoe of the abovr |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: ISI 2014 PEA Answer Key
|
Guys,
Is there a Facebook group ? Please add me to that |
«
Return to General Discussions
|
1 view|%1 views
| Free forum by Nabble | Edit this page |

