Interpreting a Confidence Interval

[jigglypuff666]

Dear peers,

I stumbled accross this question in DSE-2010, I was wondering if it could be clarified.



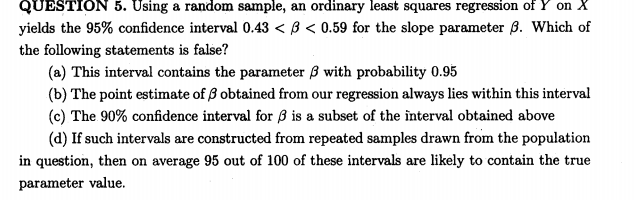
According to Prof. Goyal the answer is

"5.(a) the interval contains the parameter β with probability
0.95"

However, I think I read in Gujarati Econometrics, that

"The interpretation of this confidence interval is: Given the confi- dence coefficient of 95%, in the long run, in 95 out of 100 cases intervals like
(0.4268, 0.5914) will contain the true β2. But, as warned earlier, we cannot say that the probability is 95 percent that the specific interval (0.4268 to 0.5914) contains the true β2 because this interval is now fixed and no longer random; therefore, β2 either lies in it or does not: The probability that the specified fixed interval includes the true β2 is therefore 1 or 0." pp124

In any-case, this is an interesting debate. Takes me back to the old quantum mechanics debates- does the probability distribution exist before the measurement, or that the act of observation forces the object to "take a stand".

So, lets rephrase. After the sample has been drawn (the experiment/observation conducted), what does it mean that the population mean has 95% chance of being in that interval?

PS: Ontology is confusing :p

[Amit Goyal]

Read the question carefully. It is asked that which of the foregoing statements is FALSE. Option (a) is the correct option because statement in option (a) is false.