Problematic problems of Varian Workbook

[SINGHAM]

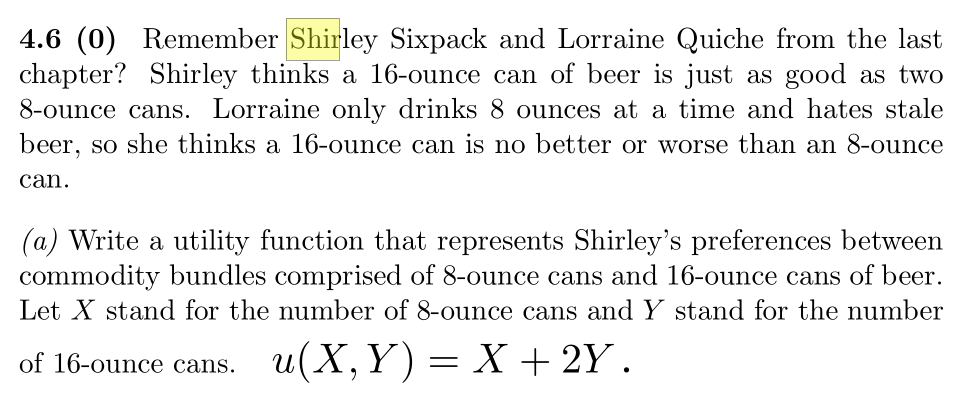Shouldn't it be instead u(X,Y ) = 2X + Y  ?

[L14]

I think its correct. Assume he is consuming 1 X and 0 Y, his utility now is 1. Now assume he is consuming 0 X and 1 Y. His utility is 2. Sounds reasonable as having one Y gives twice the utility that of X. Its a bit tricky like that of min{} ICs.
ps: I like the topic of the thread..

[SINGHAM]

Oh, Thank you so much Ben10!

[SINGHAM]

Horst and Nigel live in different countries. Possibly they have
different preferences, and certainly they face different prices. They each
consume only two goods, x and y. Horst has to pay 14 marks per unit of
x and 5 marks per unit of y. Horst spends his entire income of 167 marks
on 8 units of x and 11 units of y. Good x costs Nigel 9 quid per unit and
good y costs him 7 quid per unit. Nigel buys 10 units of x and 9 units of
y.


But, why can't we conclude that  Nigel prefers to have his own income & pries than having Horst's ? Nigel even can't afford that bundle at Horst's budget!

[Ashima]

May be the solution is:

Let Horst's bundle bE X.

Let Nigel's bundle be Y.

So if Y is preferred to X at given prices, X cannot be preferred to Y at any other price. By WARP!

What say?

[SINGHAM]

Please explain it in great detail! I am not getting!


Also, if you will please help me with this question: (Varian Workbook, Q 7.13 (e)), I will be obliged.


Why both??

[SINGHAM]

Amit Sir, please help!

Please help me with those last two questions.

[Amit Goyal]

I don't understand which two questions you want me to solve. Please post those two questions again.

[SINGHAM]

Ok, Sir!

1.Horst and Nigel live in different countries. Possibly they have
different preferences, and certainly they face different prices. They each
consume only two goods, x and y. Horst has to pay 14 marks per unit of
x and 5 marks per unit of y. Horst spends his entire income of 167 marks
on 8 units of x and 11 units of y. Good x costs Nigel 9 quid per unit and
good y costs him 7 quid per unit. Nigel buys 10 units of x and 9 units of y.


But, why can't we conclude that  Nigel prefers to have his own income & pries than having Horst's ? Nigel even can't afford that bundle at Horst's budget!




2. Varian Workbook, Q 7.13 (e)

http://discussion-forum.2150183.n2.nabble.com/file/n7586937/varian.png

Why the answer is both?

[Amit Goyal]

Since Horst's budget set is not a proper subset of Nigel's and we don't know Nigel's preferences, so there is a possibility that Nigel might like some bundle in Horst's budget set (that he can't afford in his current budget) more than the bundle of his choice from his own budget set. So, we can't tell.
For example:
Given the data: Horst chose (8, 11) from the budget 14x + 5y = 167
And Nigel chose (10, 9) from 9x +7y = 153
If Nigel's utility function is u(x, y) = 9x + 7y then he will prefer Horst's budget to his own.
And If Nigel's utility function is u(x, y) = min{9x, 10y} then he will prefer his own budget to Horst's.
 

[Amit Goyal]

7.13 Norm and Sheila consume only meat pies and beer. Meat pies
used to cost $2 each and beer was $1 per can. Their gross income used
to be $60 per week, but they had to pay an income tax of $10. Use red
ink to sketch their old budget line for meat pies and beer.
(a) They used to buy 30 cans of beer per week and spent the rest of their
income on meat pies. How many meat pies did they buy?

Ans. 10

(b) The government decided to eliminate the income tax and to put a
sales tax of $1 per can on beer, raising its price to $2 per can. Assuming
that Norm and Sheila's pre-tax income and the price of meat pies did not
change, draw their new budget line in blue ink.

Ans. Plot 2m + 2b = 60

(c) The sales tax on beer induced Norm and Sheila to reduce their beer
consumption to 20 cans per week. What happened to their consumption
of meat pies? How much revenue did this tax raise from Norm and Sheila?

Ans. m = 10, Tax Revenue = 20

(d) This part of the problem will require some careful thinking. Suppose
that instead of just taxing beer, the government decided to tax both beer
and meat pies at the same percentage rate, and suppose that the price
of beer and the price of meat pies each went up by the full amount of
the tax. The new tax rate for both goods was set high enough to raise
exactly the same amount of money from Norm and Sheila as the tax on
beer used to raise. This new tax collects how many $ for every bottle of
beer sold and how many $ for every meat pie sold? (Hint: If both goods are
taxed at the same rate, the effect is the same as an income tax.) How
large an income tax would it take to raise the same revenue as the $1 tax
on beer? Now you can figure out how big a tax on each good
is equivalent to an income tax of the amount you just found.

Ans. To get the tax revenue = 20, the beer must be taxed at $ (1/2) per can
of beer and meat must be taxed at $ 1 per meat pie (i.e. 50 % tax rate)
so that the new budget line is (1+1/2)b + (2+1)m = 60 which is equivalent to
b + 2m = 40 so that the tax revenue is 20.

(e) Use black ink to draw the budget line for Norm and Sheila that corresponds
tax in the last section. Are Norm and Sheila better off having just beer taxed
or having both beer and meat pies taxed if both sets of taxes raise the same
revenue.

Ans. To answer this, we will compare budget sets in (c) and (d).
In case of (c) the budget line is 2m + 2b = 60 and the chosen bundle is
(m, b) = (10, 20)
In case of (d) the budget line is 2m + b = 40. Clearly he can afford (m, b)
= (10, 20). So, he is definitely at least as well off as he was in case of (c).
So, having both beer and meat pies taxed is better.

[SINGHAM]

Thank you sooooo much Sir!

I have no words to thank you ! My poor English!



Sir, please help with this one, too!


When the utility function takes the quasilinear form, u(x) + m, the
area under the demand curve measures u(x), and the area under the
demand curve minus the expenditure on the other good measures u(x)+
m.

I can't understand why "the area under the
demand curve minus the expenditure on the other good measures u(x)+
m." is true! I think there should be plus, instead of minus!

[SINGHAM]

Amit Sir, please help with this one, too!


1.When the utility function takes the quasilinear form, u(x) + m, the
area under the demand curve measures u(x), and the area under the
demand curve minus the expenditure on the other good measures u(x)+
m.

I can't understand why "the area under the
demand curve minus the expenditure on the other good measures u(x)+
m." is true! I think there should be plus, instead of minus!


2. What should be the Definition of ΔCS ?

Suppose price changes from p0 to p1, so what should be ΔCS ?
Should it be
ΔCS=(net)CS(p1)-(net)CS(p0)
or
ΔCS=(net)CS(p0)-(net)CS(p1) ?

Sir, please help!


3. Consider a consumer facing the loss of an amenity or a public good
EV = Consumer’s maximum willingness to pay to keep the amenity
CV = What society must pay the consumer to make up for the loss of the amenity
The two perspectives are like different “property rights” or “entitlements”
Since the loss would leave the consumer worse off, if the amenity is a normal good, CV > EV
Conversely, can consider a situation where consumer stands to gain; then EV > CV if normal

I can't understand why CV > EV or EV > CV?

In general, if you can let me know that why this is true, i will be obliged:

In the case of a price drop or subsidy (i.e. a negative tax):
1.If the demand for good 1 is normal: CV > ∆CS > EV
2.If the demand for good 1 is inferior: : CV < ∆CS < EV

In the case of a price increase or a tax:
1.If the demand for good 1 is normal: : CV < ∆CS < EV
2.If the demand for good 1 is inferior: CV > ∆CS > EV

Unless Qusasilinear preferences, which i can understand is CV=∆CS=EV

[SINGHAM]

Amit Sir, Please help with these three!