SEQUENCE AND SERIES
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
SEQUENCE AND SERIES
|
sigma n=1 to n= infinty n!/n^n ?
Sigma n=1 to n= infinity ln n/n^2? Please help! |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: SEQUENCE AND SERIES
|
TEST WHETHER THEY CONVERGE OR DIVERGE?
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: SEQUENCE AND SERIES
|
2.Sigma n=1 to n= infinity ln n/n^2
Sum= ln 1/1 + (ln2)/ 4 .... = (ln 2)/4+ (ln 3)/9 and so on let f(x)= ln x /x^2 Since f is a monotone decreasing function and integration of ln x /x^2 from x=1 to infinity converges to 1 so the sum converges to 1 as well (by Integral test for convergence) You can check this link : https://en.wikipedia.org/wiki/Integral_test_for_convergence 1. sigma n=1 to n= infinty n!/n^n Sum= 1/1 + 2/2^2 + 3*2/ 3^3 + 4*3*2/ 4^4 and so on If we simplify a lil, Sum= 1+ 1/2 + 2/9 + 2*3/ 4^3 ..... We exclude 1 for the moment and take series 1/2 + 2/9 + 6/64... New sum = 1/2 + 2/9 + 2*3/ 4^3.... We can see T(n+1)= T(n) * (n+1/n+2)^ (n+1) from n=1 onwards So T(n) gets multiplied by (n+1/n+2)^ (n+1) to get T(n+1) Now the multiplier is a decreasing function as (x+1/x+2) ^ (x+1) is decreasing with maximum value at x=1 So T(n) * (n+1/n+2)^ (n+1)< T(n) * (4/9) So T(n+1) < T(n) * (4/9) New sum is less than a g.p series with first term 1/2 and common ratio 4/9 so new sum < 9/10 so sum < 1+ (9/10) sum < 19/10 If you find a quicker method for ques 1 please share it. |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: SEQUENCE AND SERIES
|
for question 1, Ratio test will help,
if limit |Tn+1/Tn| < 1 series converges n-infinity if we do this we get limit (n/n+1)^n which is 1/e < 1 series converges |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: SEQUENCE AND SERIES
|
Thanks
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: SEQUENCE AND SERIES
|
In reply to this post by Eco16
For 1, We can also use the nth term test.
Proving lim(n tends to infinity) a(n) =0 we can prove that the series is convergent. |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: SEQUENCE AND SERIES
|
The nth-term test for divergence is a test for the divergence of an infinite series.
Unlike stronger convergence tests, the term test cannot prove by itself that a series converges. In particular, the converse to the test is not true; instead all one can say is: 
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: SEQUENCE AND SERIES
|
thank you!!
Sigma n=1 to infinity 3n+4/2n^2+3n+5? using comparison test check if it converges or diverges. Can somebody help me with this? |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: SEQUENCE AND SERIES
|
also n=1 to infinity 3^n/2^n+3^n? comparison test!
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: SEQUENCE AND SERIES
|
In reply to this post by Eco16
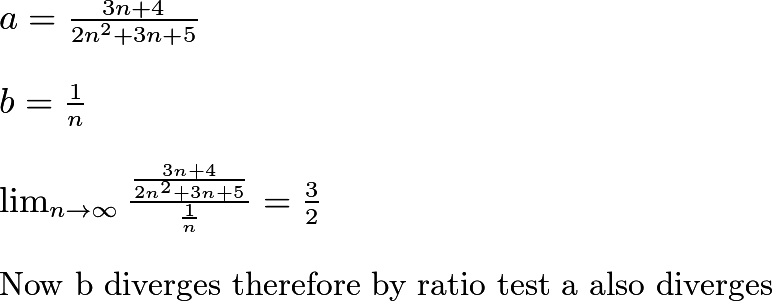
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: SEQUENCE AND SERIES
|
In reply to this post by Eco16
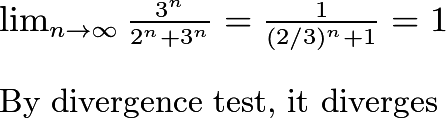
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: SEQUENCE AND SERIES
|
isn't ratio test defined as a n+1/a n?
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: SEQUENCE AND SERIES
|
This post was updated on Apr 20, 2016; 12:47pm.
In reply to this post by Eco16
I think the ratio test will fail in this question. We will have to apply Rabe's test.
PS: this is just conjecture. |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: SEQUENCE AND SERIES
|
In reply to this post by Eco16
Yeah sry. I meant the limit comparison test
 Its the one where you look at the limit for some related series which you know diverges/converges. If its a finite constant, then the series in question will do the same too. Its the one where you look at the limit for some related series which you know diverges/converges. If its a finite constant, then the series in question will do the same too.
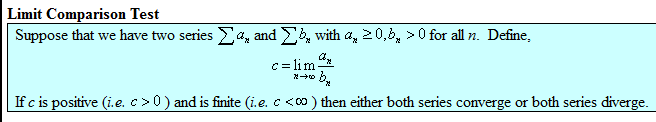 And Raabe's works too |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: SEQUENCE AND SERIES
|
In reply to this post by Dr. Strange
what is the sum of x+(1/2*X^2)+(1/3*x^3)+........?
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: SEQUENCE AND SERIES
|
-ln(1-x) has the same series expansion for x belongs to (-1,1) and infinite series
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: SEQUENCE AND SERIES
|
Thanks :)
|
«
Return to General Discussions
|
1 view|%1 views
| Free forum by Nabble | Edit this page |

