Strong vs Weak Pareto Efficiency - May 23
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Strong vs Weak Pareto Efficiency - May 23
|
Administrator
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Strong vs Weak Pareto Efficiency - May 23
|
I got,
Strongly Pareto Efficient Allocations: (x1,y1) (x2,y2) (0,2) (1,0) (1,0) (0,2) Weakly Pareto Efficient Allocations: All allocations where either x1 or y1 is discrete! Example: (0,y1) (1, 2-y1) (1,y1) (0, 2-y1) (x1,0) (1-x1, 2) (x1,1) (1-x1, 1) (x1,2) (1-x1, 0) |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Strong vs Weak Pareto Efficiency - May 23
|
@Sir,
Please reply if its wrong... |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Strong vs Weak Pareto Efficiency - May 23
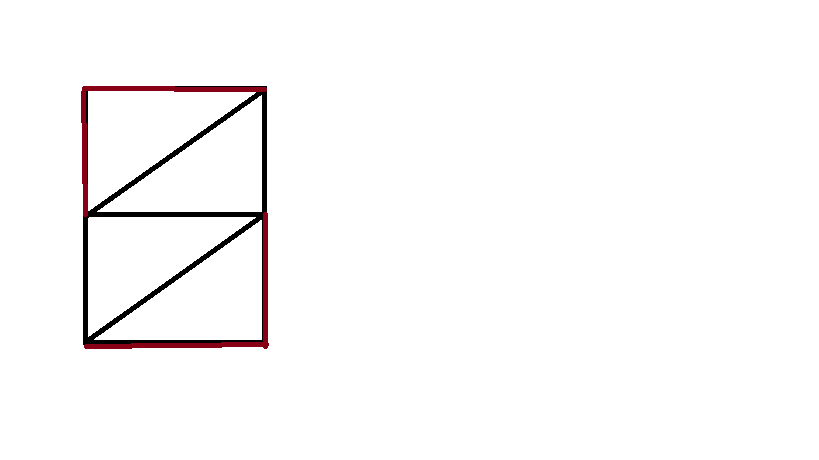 the set of strongly efficient allocations is the same as what AJ got, and the set of weakly efficient allocations consists of all the points that i've highlighted in red (including the end points). i'm not sure about this! |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Strong vs Weak Pareto Efficiency - May 23
|
In reply to this post by AJ
Stong pareto allocations - same as AJ
Weak pareto allocations - (a,0) and (1-a,2) ; (1,a) and (0,2-a) where a belongs to (0,1) |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Strong vs Weak Pareto Efficiency - May 23
|
Administrator
|
In reply to this post by anon_econ
AJ, Aditi and Vasudha, All of you got strongly Pareto efficient allocations correct. But think about weakly pareto efficient allocations again. For example:- check that ((0.5, 1), (0.5, 1)) is weakly efficient but is not included in the set you illustrated.
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Strong vs Weak Pareto Efficiency - May 23
|
@Amit Sir,
It is included in the set I wrote... .. "all those allocations where either X1 or Y1 is discrete".... Can u give an allocation, where both goods are not discrete and still its weakly pareto efficient.. So, that I can compare it with my answer..and find the mistake... |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Strong vs Weak Pareto Efficiency - May 23
|
Sir a weak pareto efficient allocation is one that makes only one agent better off naa? (without making both better off..) So can't any allocation like (a,b) and (1-a, 2-b) for a,b belonging to (0,1) would be weakly PO? Since their endowments haven't been mentioned..
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Strong vs Weak Pareto Efficiency - May 23
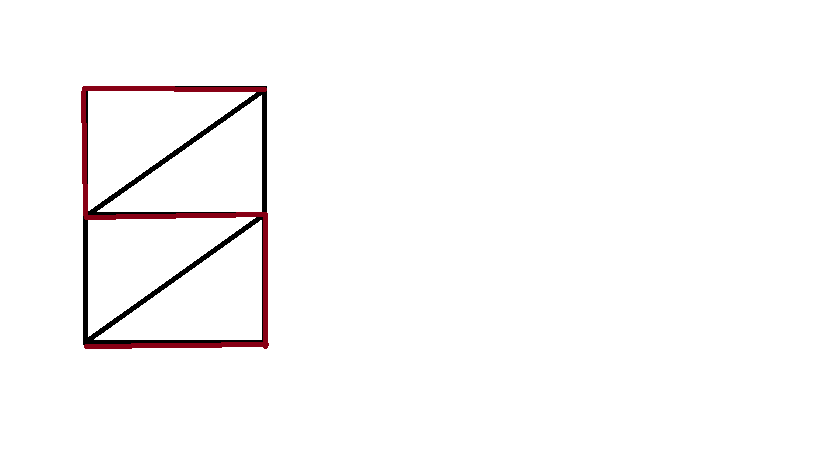 what about this? |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Strong vs Weak Pareto Efficiency - May 23
|
Administrator
|
Thats right Vasudha. Well done.
Let F denotes set of all feasible allocations (set of all allocations in the edgeworth box) Let x(i) denotes i's consumption of good x, and y(i) denotes i's consumption of good y Set of weakly efficient allocations is given by {((x(1),y(1)),(x(2),y(2))) ∈ F| y(1) = 2} U {((x(1),y(1)),(x(2),y(2))) ∈ F| y(2) = 2} U {((x(1),y(1)),(x(2),y(2))) ∈ F| max (x(1),y(1)) = 1} U {((x(1),y(1)),(x(2),y(2))) ∈ F| max (x(2),y(2)) = 1} |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Strong vs Weak Pareto Efficiency - May 23
|
Administrator
|
In reply to this post by AJ
Hi AJ Thats not the precise description of weakly efficient set. Consider an allocation
((1,1.5), (0,0.5)) This is part of the set you listed but is not weakly efficient as ((0,2), (1,0)) beats it. |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Strong vs Weak Pareto Efficiency - May 23
|
Sir I think my GE concepts are terrible. Please recommend where to study it from. First time round, I studied it from HRV. But I need more practice on such questions. I keep getting confused...
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Strong vs Weak Pareto Efficiency - May 23
|
okay.. This means I also took lower left and upper right vertical lines of vasudha's edgeworth box too..which are infact not weakly pareto optimal..!! :(
@ AMIT sir, vasudha what is the best way to proceed in G.E. questions..?? like for competitive Equilibrium.. I start with demand functions of both individuals and then take one good.. and equal the demand with endowment of that good.. (and normalize one of the price) And for Pareto optimal.. I take some random points, see their behavior.. and draw conclusion on that basis... but this way ofcourse I can make mistakes like above... So, is it always better to try to find points on edgeworth box...like vasudha did.. and then draw conclusion... Vasudha, how u proceed for these questions...?? |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Strong vs Weak Pareto Efficiency - May 23
|
Administrator
|
In reply to this post by aditi5000
Hi Aditi,
A very good reference for this topic is Ch-15 of Microeconomic Theory by Mascollel, Whinston and Green But it would be better if you do Ch 1, 2 and 3 of the same before doing chapter 15. The book is rigorous, and extremely clear in its presentation. If you want slightly less rigorous treatment, Ch - 17 of Microeconomic Analysis by Varian could be a reasonable choice. |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Strong vs Weak Pareto Efficiency - May 23
|
Administrator
|
In reply to this post by AJ
AJ, The "best" way varies with the problem. The way you listed is good and will work for all the questions provided you are careful while doing computations but there are usually multiple ways to solve the same problem. And the best is learned through experimentation and practice.
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Strong vs Weak Pareto Efficiency - May 23
|
@Sir,
Okay, I will see the above mentioned book too.. Sir it will be really helpful if you can see my following solution for GE from 2006 DSE paper.. Please.. And tell me if there is any mistake in my method or "Concepts" most importantly... I know I am bugging U a lot, but I haven't studied GE in my course as such and I always make mistake in 1st go.. I want to attempt those 3 questions right which we always get in DSE.  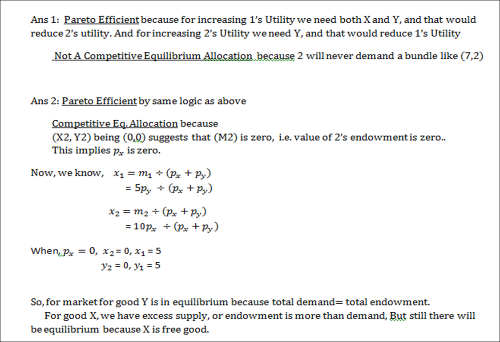 
|
«
Return to General Discussions
|
1 view|%1 views
| Free forum by Nabble | Edit this page |

