maths
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
maths
|
Suppose n>=9 is an integer. Let u= n^1/2 + n^1/3 + n ^1/4.
Then which of the following relationships between n and u is correct? A. n=u B. n>u C. n<u D. none of the above. Also, I have a doubt in dse 2008 question 55. http://economicsentrance.weebly.com/uploads/1/1/0/5/1105777/28_june_2008_option_a.pdf I'm getting the limit to be 0 for all x other than 0, and infinity for x=0. Plz confirm this. And if this is correct then is the answer d? I mean if a sequence has an infinite limit do we say the limit does not exist? |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: maths
|
I am getting these answers...
for first question: u= n^1/2(1+1/n^1/6+1/n^1/4) .... i took n^1/2 common ... then for values small values like 9 or 10 ... this is ofcourse less than n... for larger values... the bracketed expression is 1+ something .. its less than 2 always... so at max.. u = 2.n^1/2.. i.e. (twice of root n)... for all values above 4.. twice of root of a number is less than the no.. so, B. n>u (thought after typing this, m feeling m wrong.. :p ) for second question: What does this notation [0,1/n] ..in base of function g ..means...??? |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: maths
|
This post was updated on May 04, 2012; 8:00pm.
In reply to this post by anon_econ
AJ's solution is acceptable, of course, but a slightly different approach will make our lives even simpler. Notice that both n^(1/6) and n^(1/4) are greater than 1 for n > 1. So, their reciprocals will be less than 1. In symbols, u = n^(1/2) + n^(1/3) + n ^(1/4) < 3n^(1/2). Hence for n >= 9, u < n.
As for the DSE question, fn = n if x belongs to [0,1/n], and 0 otherwise. Limit fn as n tends to infinity exists everywhere except at x = 0. So, you're right, the answer should be (d). |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: maths
|
from dskul..2009..
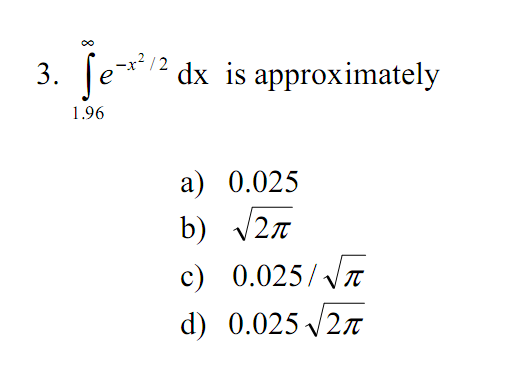 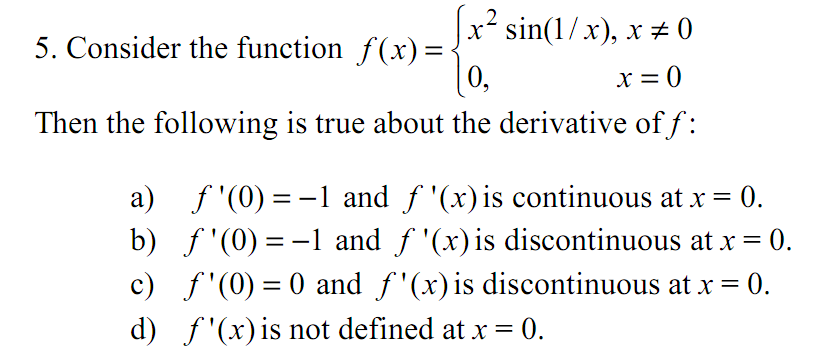 |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: maths
|
This post was updated on Jun 19, 2012; 12:20pm.
Answer to the second question should be (c). It follows directly from the definition of derivative of a function.
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: maths
|
I keep on having trouble with derivative.. plss explain this... see...
f'(x)= 2xsin(1/x)-cos(1/x) , if x is not equal to zero and 0 , if x is equal to zero... is this right?? ok, now.. f'(0)=0.. that's fine... but how do we find the limits of f'(x) when x approaches zero... to check for continuity.. can u please explain me continuity of this sin(1/x) kinda function.. i dont get this.... |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: maths
|
hi AJ....U CAN DO FIRST QUESTION LIKE THIS....
WE KNOW THAT THE FUNCTION OF "STANDARD" NORMAL DEVIATE ie.Z IS 1/root 2 pie.e^(-x^2/2)...{check any normal stats book for this function if u cant read it properly} now try to imagine a standard normal curve.....area covered btwn (-1.96,1.96) is 95% or 0.95.... this means we are left with 0.05 area of which 0.025 each lies to the left and right of(+- 1.96) now we want area from 1.96 till infinity..... integral of {1/ root 2 pie .e^-x^2/2} over (1.96, infinity) =0.025 we can take out constant 1/root2 pie....with the desired integral in brackets....taking constant other side u get answer as 0.025(under root 2 pie)....ie. option d i kno its quite messy ....sry dnt have access to this graph technology...:P |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: maths
|
Thanks guys

|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: maths
|
In reply to this post by AJ
AJ, first, an observation: having confidently arrived at f'(0) = 0, do you need to check for the second part? :)
More seriously, having found out f'(x), when you apply the limit "as x tends to zero" to the expression, you'll find that the limit does not exist. Hence, the discontinuity at x = 0. Limit of sin(1/x) as x approaches zero does not exist. You can, however, evaluate the limit of xsin(1/x) as x approaches zero by Sandwich theorem. Ritu, it's not messy at all! I must admit it did not strike me immediately. Great job! 
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: maths
|
@chocolate frog... thanks..
@ ritu... Neat..!!! |
«
Return to General Discussions
|
1 view|%1 views
| Free forum by Nabble | Edit this page |

