DSE 2007
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
DSE 2007
|
Can someone please help me out with question 38? As per my reasoning I think it should be 'b' but the answer key says 'a'.
Thanks. :) 
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2007
|
Simplifying the utility function as a Cobb-Douglas function, we have -
U(c1,c2) = c1*(c2)^(1/(1+∂)). The budget constraint is c1 + c2/(1+r) = w1 + w2/(1+r). Solving, we get c1 = (w1 + w2/(1+r))/(1+(1/(1+∂))). Now, if c1 increases, the borrowing increases. c1 increases if ∂ increases. Thus the answer is (a). |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2007
|
In reply to this post by NM
Since optimal borrowing, b = [(1+§)*(w1*(1+r) + w2)/(2+§)*(1+r)] - w1
differentiating wrt § yields [(w1*(1+r)+w2)/(1+r)*(2+§)²] is positive, thus b increases! |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2007
|
Gayyam..How did you get the cobb douglas version?
@drefus..how did you find optimal borrowing?
MA Economics
DSE 2014-16 |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2007
|
The given utility function is monotonically transformed version of Cobb Douglas function.
The optimal borrowing in period 1, b = C1* - W1 Where C1* is optimal consumption level in period 1 |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2007
|
In reply to this post by SoniaKapoor
Since utility functions are the same up to a monotonic transformation, you can choose to transform any utility function as you like with a suitable monotonic transformation. (DSE 2008 q31, q32 are good examples)
Often, this simplifies calculations. e.g. here, we have U(c1,c2) = log(c1) + log(c2)/(1+∂) Using the properties of log (log(a) + log(b) = log (a*b)), we get U(c1,c2) = log (.c1*(c2)^(1/(1+∂))) But, y=e^x is a monotonic transformation. So, e^U(c1,c2) is equivalent to the utility function, which is c1*(c2)^(1/(1+∂)). Do let me know if this is clear. |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2007
|
Thank you so much! :D
I didn't think of converting the utility function into Cobb-Douglas. 
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2007
|
In reply to this post by Gayyam
gayyam..Thanx I got that
 Bt i still didnt get how u got c1 value?Please help.I'm stuck.
MA Economics
DSE 2014-16 |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2007
|
This is my approach and I'm getting the answer
Qn 36: MU1=1/c1 MU2=1/(c2.(1+d)) MRS=(c2.(1+d))/c1 Slope of the intertemporal budget line is (1+r) So the optimal choice condition is (c2.(1+d))/c1=(1+r) => c1/c2= (1+d)/(1+r) Since the endowment(wage) is also a possible consumption bundle, when he borrows c1/c2 will be greater than w1/w2 ie. (1+d)/(1+r) > w1/w2 (1+d)*w2 > w1*(1+r) Qn 37: c1/c2= (1+d)/(1+r) as d increases c1 increases, he borrows more |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2007
|
In reply to this post by NM
guys pls explain 44,45,56. thanks.
 |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2007
|
@phelps
57 The standard error is given by root(p*(1-p)/n) where p=215/400, 1-p=185/400 , n=400 put in above formula u'll get it .025 :) |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2007
|
thank u Ron, can u tell how we arrive at this formula.
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2007
|
This is the formula for a proportion value.
Refer any stats book degroot , or freund..any..u'll get it.Cheers!! |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2007
|
In reply to this post by phelps.phan
For ques 44 and 45 the following rules are used
rule 1: intersection of an open set is always a closed set hence 44 ans is d rule 2: union of a closed set is always an open set hence 45 ans is c .... |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2007
|
In reply to this post by Gayyam
@Gayyam , could you please explain ques 37 ?
"Once you eliminate the impossible, whatever remains, no matter how improbable, must be the truth."
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2007
|
In reply to this post by NM
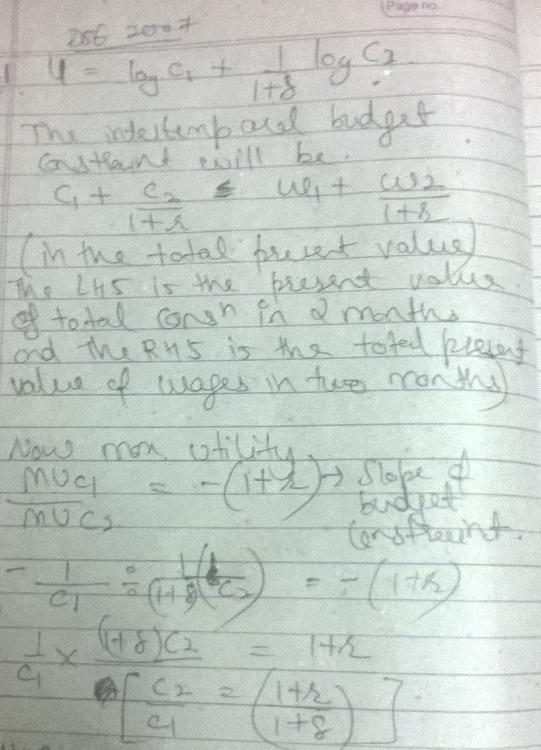 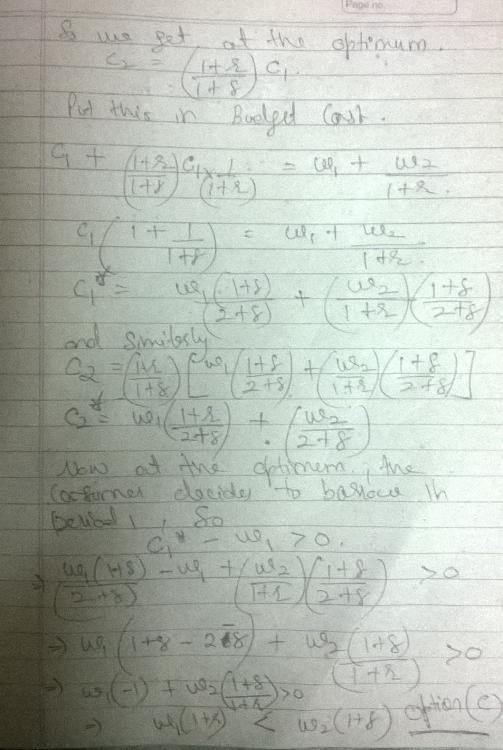 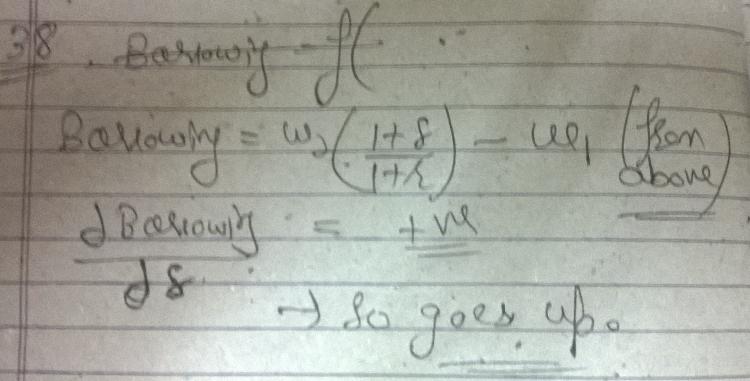
Akshay Jain
Masters in Economics Delhi School of Economics 2013-15 |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: DSE 2007
|
Thanks Akshay !!
 
"Once you eliminate the impossible, whatever remains, no matter how improbable, must be the truth."
|
«
Return to General Discussions
|
1 view|%1 views
| Free forum by Nabble | Edit this page |

