ISI Interview Question

[happy]

Anyone plz

[Algaeconomics]

Hey do you understand the meaning of concave increasing??

[Asd1995]

probably convex ICs and positive marginal utilities.

[Asd1995]

Perhaps the question is incomplete. If I assume a Rawlsian welfare function I'll get a different result than I'll  get from maximising sum of utilities which would be different from weighted sum of utilities.

The only other thing I can think of is he may want the allocation to be fair i.e no one envies each other and locations are pareto optimal.

If Utility is convex analysis for each kind of welfare function changes but again idk.

[happy]

concave increasing means utility of x increases at decreasing rate

An example of concave increasing function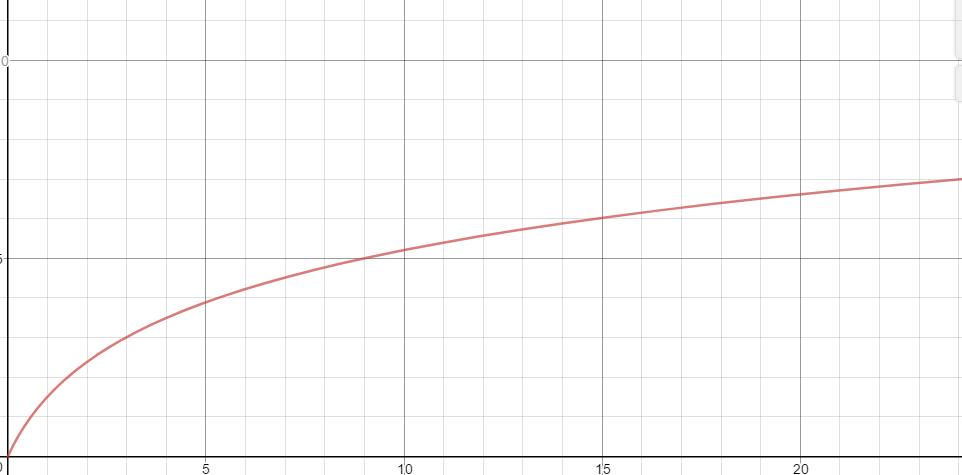



X-Axis = qty of x
Y-Axix = U(x)

[Econ17]

CONTENTS DELETED

The author has deleted this message.

[Asd1995]

If you're preparing for ISI interview, they scrapped asking math questions last year.

ANnyway,

If a polynomial with real coefficients has 1 real root, then imaginary roots occur in conjugate pairs (a+bi,a-bi)

Since this has a degree of 2, it must have both roots real. Discriminant (b^2-4ac) >=0

so A^2-4>=0 or A belongs to the set of all real values except from -2 to 2.

So permitted values in our set are [2,5] so success= 0.6

Probability Mr. Y picks out something from [2,5]= F(5)-F(2) where F is cdf of the distribution.

First I believe we have to normalise the pdf here, so that INTERGRAL f for all values of a=1

so f(a)=2a/25

F(a)= a^/25

So success= 1-4/25=21/25

[Econ17]

CONTENTS DELETED

The author has deleted this message.

[Econ17]

CONTENTS DELETED

The author has deleted this message.

[happy]

last year they asked 2 micro question or just 1?

[Asd1995]

One

[Asd1995]

Which question?

[Asd1995]

So after a bit of thought, here's my two cents;

Since we dont have a defined welfare function, we know that if preferences are convex, there exists an allocation which is fair and pareto optimal (Hal Varian). If everyone has the same preferences, the only such allocation is where everyone has the same utility.

Now if transfer from c1 to c2 results in a loss, and we still want to ensure fairness, we still need to give everyone the same bundle since everyone still has the same preference. So both cities will have same bundle, but this will not maximize total utility. Still, it is a fair and a pareto efficient outcome.

If preferences are concave a fair and PE allocation may not exist, it would depend on the nature of the function.

[Econ17]

CONTENTS DELETED

The author has deleted this message.

[Asd1995]

Sure, happy to help. Obviously I may be wrong so keep that in mind.

[ElPsyCongroo]

If they scrapped math questions then what do they ask?

One micro and one macro?

Or just one micro?

[Asd1995]

Only micro last year

[ElPsyCongroo]

What? Like one micro question?

Sure hope they don't flip the pattern and ask one math question this year :S

[Asd1995]

Hahaha micro will always be there. Math or no math can't say.

[Econ17]

CONTENTS DELETED

The author has deleted this message.