JNU ECOM 2010 ques 41-50
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
JNU ECOM 2010 ques 41-50
|
This post was updated on Mar 01, 2014; 6:08pm.
Sorry guys. Due to some reason i couldnt post questions for the last two days. neways m back now
 QUES41 Which of the following is a function? a. A rule that assigns the circumference of a rectangle to its area. b. A rule that assigns to each number its square root. c. A rule that assigns to each person in a classroom his/her height. d. A rule that assigns the salary of a person to his/her years of education. Answer-c QUES 42-45 Consider a country in which there are four different types of people Red, Blue, Green and yellow. All Reds earn the same income. The same is true about Blues, Greens & yellows. However, the Red income, the blue income, the green income & yellow income can be different from each other. A distribution of income of a country i given by Di specifies the Red income, Blue income, Green income & Yellow income & also the number of Reds, blues, Greens and yellows. the following table gives the possible income distribution for the country: 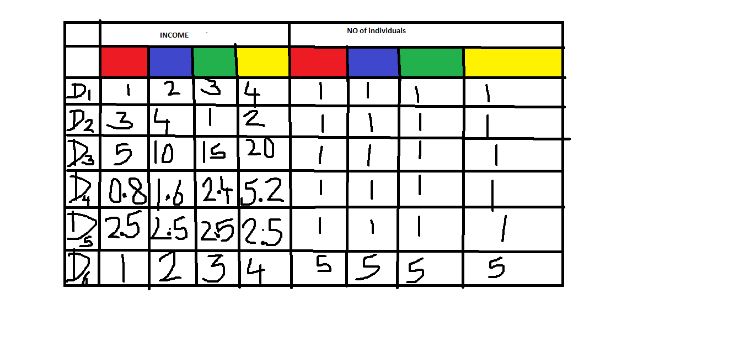 QUES 42 Let Gi denote the Ginni coefficient for the income distribution i. Which of the following is true? 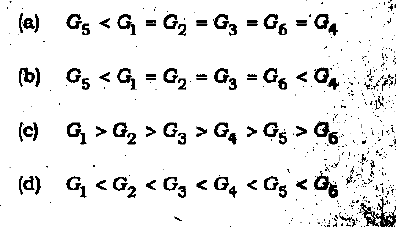 Aswer - b QUES43 Let sigma i denote the standard deviation for the income distribution i. Which of the following is true? 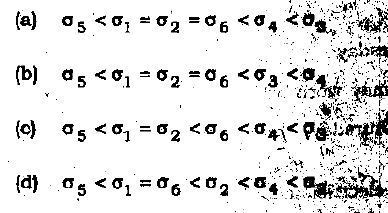 Answer- a 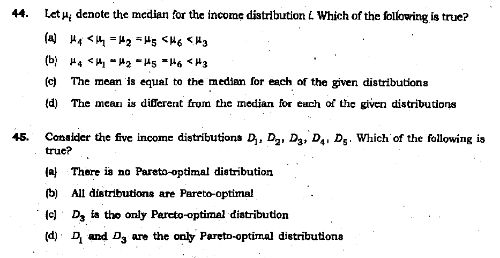 Answer 44- b  Answer-b QUES 47 In case the painter works on Friday, which among the following statements must be untrue? a. The carpenter may work on Wednesday. b. The carpenter & electrician may work on consecutive days. c. In case, the carpenter works on Thursday , the electrician has to work on the previous day, i.e Wednesday d. The plumber may work before the electrician does. Answer-c QUES48 Which arrangement among the following is possible? a.The painter will work on Wednesday & the plumber on Thursday. b.The carpenter will work on Tuesday & the painter on Friday. c.The painter will work on Monday & the carpenter on Thursday. d.The carpenter will work on Wednesday & the plumber on Thursday. Answer-d QUES 49 There are two egg delivery boys you can order eggs from . The probability of the first boy falling & breaking all the eggs is 1/2 and the probability of second boy falling & breaking all the eggs is 1/5. How would you distribute your order for eggs so as to minimize expected loss of eggs? a. Order all your eggs from the first boy. b. Order all your eggs from the second boy. c. Distribute the order for eggs between the two boys equally. d. Order three-fourths of your eggs from first boy & the rest from the second boy. Answer QUES50 If you integrate the function f(x)=1/x from 1 to 3, you get: a. 2 b. log3 c. log4 None of the above Answer-b |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: JNU ECOM 2010 ques 41-50
|
How to do 49?
And for 45 i think the answer is part a. No idea please help with these two n confrm the rest |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: JNU ECOM 2010 ques 41-50
|
Let the total no of eggs to be ordered be 100..let us give X to boy 1 and 100-x to boy 2
Let Y be the no of eggs we ultimately get E(Y)= 0.5*x+0*x+ 0.8*(100-x)+0*(100-x) E(y)= 80-0.3x Now we will seek to maximize Y. It will be max if x=0. So we will place the entire order on boy 2 |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: JNU ECOM 2010 ques 41-50
|
In reply to this post by Arushi :))
Hi Arushi
Could you explain me questions 42-25... |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: JNU ECOM 2010 ques 41-50
|
This post was updated on Mar 01, 2014; 5:55pm.
In reply to this post by Arushi :))
Thanks kangkan..:)
hi ron for these questions u should be having knowledge of ginni coefficient & measures of central tendency For ques 42 for finding out ginni coefficient we have to take absolute deviation of incomes pairwise , i.e difference of each term with the other , add them up. and then we divide it by 2*mean income*n^2 example for D1 consider red people deviation would be(absolute) 1-2 = 1, 1-3 = 2 1-4 = 3 then for blue people : 2-1=1 ,2-3=1, 2-4=2 then for green, 3-1=2 , 3-2=1, 3-4=1 then for yellow 4-1=3, 4-2=2, 4-3=1 next we have to add all these deviations and divide by 2n^2* mean income mean income is 10/4=2.5 n^2= 16 n sum of deviations is 20 so ginni coeff would be 0.5 the no. of people n incomes are same in country 2 .. thus G1= G2 then option c & d ruled out only comparison between country 4 & 6 is left coming to country 6 the sum of deviations is 20 but there are 5 people of each kind so we will multiply it by 5 = 100. then mean income is 50/20= 2.5 and n^2= 400 so G6 would be 0.1 and lastly for country 4 calculating will give sum of deviations as 28 n^2 = 16 mean income = 2.5 G4= 0.7 G4>G6 Hence option b |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: JNU ECOM 2010 ques 41-50
|
This post was updated on Mar 01, 2014; 6:11pm.
Ques 43
using the formula of standard deviation and taking each country as different distribution.... we get same std deviation for country 1 , 2, 6 =1.1 country 4 ' s standard deviation is 1.6 and country 3's standard deviation is 5.5 so ans would be part a.. |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: JNU ECOM 2010 ques 41-50
|
For ques 44..
the mean income for country 1, 5 ,6 2 is same 10/4 = 2.5 median is 5/2 for all of them = 2.5 for country 4 mean income is 2.5 and median is 2 so option c & d are ruled out.. for country 3 the median is 25/2 = 12.5 so country 4 has smallest median and country 6 has largest .. rest have the same so option b |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: JNU ECOM 2010 ques 41-50
|
45 i am not getting.
its not given whether people of a color can use an income of different color or not. if they can use then , all of them are Pareto optimal because giving one's income to other would make one better off & other worse off. But if you give blue income to yellow person and he can't use then he isn't made better off.so i dunno.someone help.. |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: JNU ECOM 2010 ques 41-50
|
In reply to this post by Arushi :))
Thank you Arushi :))Really appreciate
 Please tell me Which book should i refer to for such questions and gini coefficient? |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: JNU ECOM 2010 ques 41-50
|
hehe.. u are welcome ron
 n u can refer to the book on development economics - debraj ray . this is the book we had in third year |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: JNU ECOM 2010 ques 41-50
|
Hi
Where can i get this question paper |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: JNU ECOM 2010 ques 41-50
|
Hi shietal,
the question papers are available on this site itself on the Lessons page. |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: JNU ECOM 2010 ques 41-50
|
In reply to this post by Arushi :))
Arushi, I think the answer is (b) - all alocations are pareto optimal, because to move away from any of this given distributions you would have to take away some income (say z) of atleast 1 person (let that be an arbitary person i). Irrespective of who you give this z to, and whether or nnot they can use it, i will be made worse off. Therefore every allocation is pareto optimal.
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: JNU ECOM 2010 ques 41-50
|
In reply to this post by Arushi :))
@Arushi , how have you calculated the median for this gini coefficient question ? Sum of middle incomes /2 ?
Don't we have to take their respective frequencies into account ?
"Once you eliminate the impossible, whatever remains, no matter how improbable, must be the truth."
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: JNU ECOM 2010 ques 41-50
|
IN THE FORMULA THAT YOU HAVE GIVEN, HAVE YOU DIVIDED THE NUMERATOR BY 2?
|
«
Return to General Discussions
|
1 view|%1 views
| Free forum by Nabble | Edit this page |

