JNU ECOM 2011 QUES 1-10
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
JNU ECOM 2011 QUES 1-10
|
QUES 1
Suppose two fair, six-sided dice are rolled. The probability of obtaining a value from the first dice that is at least two greater than the value from the second dice is:  Answer-c QUES 2 If the radius of a circle is increased by 20%, then the area is increased by:  Answer-a QUES 3 Let x, y and z be distinct integers. x and y are odd and positive , and z is even and positive. Which of the following statements cannot be true? 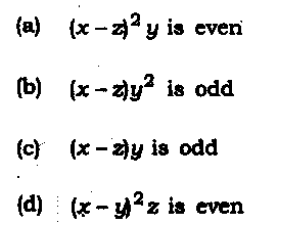 Answer-a QUES 4 A line that is 13 units long has (4,1) as one of the endpoints. Which of the following could be the other endpoint?  Answer-a QUES 5 If p & q are the roots of the equation x^2-bx+c=0, then what is the equation if the roots are (pq+p+q) and (pq-p-q)? 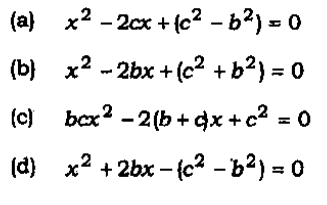 Answer-a QUES 6  Answer-b QUES 7 Suppose we know that |a|< 3. Which of the following is enough to imply that |b|<5 ?  Answer-c QUES 8 A population consists of the following seven numbers: 2003, 1999, 2001, 1997, 2000, 2005, 1995 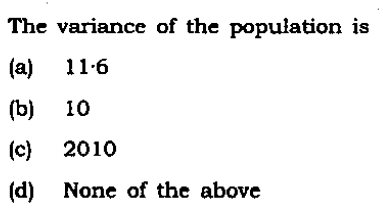 Answer-b QUES 9 Suppose a person's utility is given by u(x,y). If good x and good y are perfect substitutes, then the indifference curves are:  Answer-a QUES 10  Answer-c |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: JNU ECOM 2011 QUES 1-10
|
Hey Arushi
The answer for quest 6 is a... (x+7)>0 so x>-7
MA Economics
DSE 2014-16 |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: JNU ECOM 2011 QUES 1-10
|
In reply to this post by Arushi :))
hey can u plz tell how to do question no. 7.
my answer is (a). plz help. thanx |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: JNU ECOM 2011 QUES 1-10
|
This post was updated on Mar 07, 2014; 9:58am.
ooopssssss. i did that by mistake yeah a is right for sixth . Thanks Sonia.
For ques 7, Hi Sandeep 'a' can't be the answer, |a+b|<8 => -8< (a+b) < 8 => -8-a < b < 8-a Now, its given that |a|<3, => -3< a <3 If i pick 2.5 as a, then, i would get -10.5<b< 5.5 And we need |b|< 5, i.e -5<b<5 So we are getting a contradiction here. I chose |a-b| <,= 2 because -2 <,= (a-b) <,= 2 -2-a < -b < 2-a ( not strict inequality ) a+2 > b > a-2 a-2 < b < a+2 Now, if I choose any -3 < a < 3 I will always get |b| < 5. ( you can check by taking numbers) |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: JNU ECOM 2011 QUES 1-10
|
Thank you so much

|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: JNU ECOM 2011 QUES 1-10
|
In reply to this post by Arushi :))
can u plz tell how to do question no. 4?????
my answer is (c). kindly help |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: JNU ECOM 2011 QUES 1-10
|
The formula to find length of a line given its two end points is:
underroot of this: (x1-y1)^2+(x2-y2)^2 Where (x1,x2) & (y1,y2) are the two endpoints of the line. If you take c as the option , the two end points will be, (4,1) & (3,7) This will fetch you (1+36)^1/2 as the length . underroot 37. So, this can't be the right answer. Taking (4,1) & (-1,13) gives (25+144)^1/2 = (169)^1/2 = 13 Which agrees with the given length of 13 units. So (a) is the right option. 
|
«
Return to General Discussions
|
1 view|%1 views
| Free forum by Nabble | Edit this page |

