Maths ! help !
12
 Apr 19, 2014; 4:38am
Apr 19, 2014; 4:38am
 Apr 19, 2014; 4:45am
Apr 19, 2014; 4:45am
 Apr 19, 2014; 4:46am
Apr 19, 2014; 4:46am
 Apr 19, 2014; 4:47am
Apr 19, 2014; 4:47am
 Apr 19, 2014; 6:22am
Apr 19, 2014; 6:22am
 Apr 19, 2014; 6:29am
Apr 19, 2014; 6:29am
 Apr 19, 2014; 6:32am
Apr 19, 2014; 6:32am
 Apr 19, 2014; 6:35am
Apr 19, 2014; 6:35am
 Apr 19, 2014; 7:17am
Apr 19, 2014; 7:17am
 Apr 19, 2014; 7:54am
Apr 19, 2014; 7:54am
 Apr 19, 2014; 8:01am
Apr 19, 2014; 8:01am
 Apr 19, 2014; 8:03am
Apr 19, 2014; 8:03am
 Apr 19, 2014; 8:14am
Apr 19, 2014; 8:14am
 Apr 19, 2014; 8:17am
Apr 19, 2014; 8:17am
 Apr 19, 2014; 8:21am
Apr 19, 2014; 8:21am
 Apr 19, 2014; 8:25am
Apr 19, 2014; 8:25am
 Apr 19, 2014; 8:54am
Apr 19, 2014; 8:54am
 Apr 19, 2014; 9:00am
Apr 19, 2014; 9:00am
 Apr 19, 2014; 9:04am
Apr 19, 2014; 9:04am
 Apr 19, 2014; 9:07am
Apr 19, 2014; 9:07am
12
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Maths ! help !
|
1. The condition that the straight line y=mx+c may touch the hyperbola (x^2/a^2) - (y^2/b^2)=1 is
C^2= a^2m^2-b^2 C^2=a^2m^2+b^2 C^2=m^2-a^2b^2 C=b^2m^2-a^2
"Once you eliminate the impossible, whatever remains, no matter how improbable, must be the truth."
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Maths ! help !
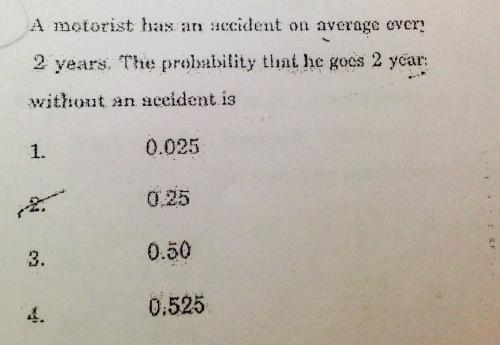
"Once you eliminate the impossible, whatever remains, no matter how improbable, must be the truth."
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Maths ! help !
|
In reply to this post by Anjali
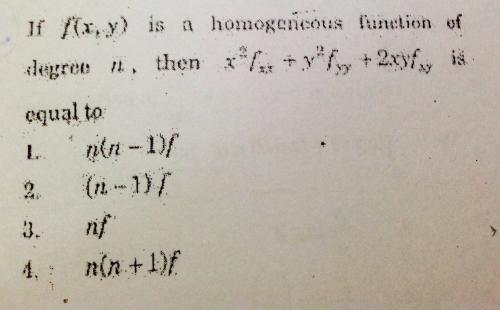
"Once you eliminate the impossible, whatever remains, no matter how improbable, must be the truth."
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Maths ! help !
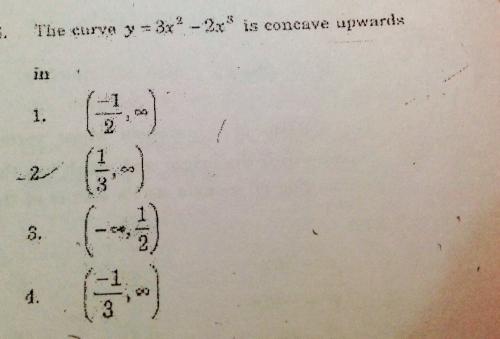
"Once you eliminate the impossible, whatever remains, no matter how improbable, must be the truth."
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Maths ! help !
|
I am trying to solve. Meanwhile can you tell me these are important for which exam?
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Maths ! help !
|
This post was updated on Apr 19, 2014; 6:36am.
In reply to this post by Anjali
Q1) a.
Q2) 0.25 (since probability of accident in a particular year is 0.5, the probability of non occurance of accident in a particular year is 0.5..so probability of non occurance of accident in both the years is 0.5*0.5=0.25). Q4) c
"I don't ride side-saddle. I'm as straight as a submarine"
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Maths ! help !
|
Q3) I think you can use Euler's theorem and try...
"I don't ride side-saddle. I'm as straight as a submarine"
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Maths ! help !
|
In reply to this post by Anjali
2b
4 c |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Maths ! help !
|
In reply to this post by Anjali
(-infinity, 1/2)
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Maths ! help !
|
In reply to this post by Granpa Simpson
How did you take 0.5 as the prob of accident in a year? Its nowhere given that in a year either an accident will take place or won't take place.
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Maths ! help !
|
I think becaz there can be only two outcomes..accident or not accident...so we take each with equal prob 1/2..maybe
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Maths ! help !
|
In reply to this post by Anjali
Yes i agree with ron , prob should be half for each.
I forgot Euler's theorem.. nyone??? |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Maths ! help !
|
In reply to this post by The Villain
In the same ques, had it been asked prob of two accidents the? Again it would be 0.5x0.5! Not a correct logic i feel :(
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Maths ! help !
|
assuming equally likely events probability of occurance of accident in a year is 0.5...
"I don't ride side-saddle. I'm as straight as a submarine"
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Maths ! help !
|
In reply to this post by Shefali
Eulers Theorem states that for a homogeneous equation of degree n,
nf(x,y)= x*fx + y*fy where fx and fy are the partial derivatives wrt x and y respectively..!!!!
"I don't ride side-saddle. I'm as straight as a submarine"
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Maths ! help !
|
In reply to this post by Anjali
Its n(n-1)f...
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Maths ! help !
|
In reply to this post by Anjali
By using Euler’s Theorem, n*f(x,y)=x*fx+y*fy
Again differentiating partially wrt x and y respectively we get, Or, n*fx = fx + x*fxx + y*fyx...................(1) Or, n*fy = fy + y*fyy + x*fxy………………...(2) By using Young’s Theorem fxy = fyx. Multiplying (1) by x, (2) by y and adding and rearranging we get. n*(x*fx + y*fy)= (x^2)*fxx+ (y)^2*fyy+ 2*x*y*fxy + (x*fx+y*fy) Substituting x*fx+y*fy = nf we get, n*n*f - n*f = (x^2)*fxx+ (y)^2*fyy+ 2*x*y*fxy . or, (x^2)*fxx+ (y)^2*fyy+ 2*x*y*fxy = n*f*(n-1)
"I don't ride side-saddle. I'm as straight as a submarine"
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Maths ! help !
|
In reply to this post by Ashima
Consider two equally likely events A & B s.t.
A= accident occurs in the first year. B= accident occurs in the second year. Also AUB= S Now A and B are mutually exclusive as if accident occurs in year 1 then it cannot occur in year 2 and vice versa. Hence occurance of one event prevents occurance of the other hence mutually exclusive. Now P(AUB) = P(A)+P(B) again 1= P(A)+P(B), if A and B are equally likely then P(A)=P(B)=1/2 Using this you will get the answer as 0.25.
"I don't ride side-saddle. I'm as straight as a submarine"
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Maths ! help !
|
In reply to this post by Granpa Simpson
Subhayu can you explain 4 ?
For upwards , the 1st derivative must be greater than 0 Which concludes x<1 Now for concave second derivative must be less than 0 Which concludes x>1/2 Iam stuck here Please help And for that Euler's theorem I have taken a function (xy)^n and then I have substituted its partial derivatives in the given equation. Am I going right ?
"Once you eliminate the impossible, whatever remains, no matter how improbable, must be the truth."
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: Maths ! help !
|
In reply to this post by Granpa Simpson
Awesome subhayu !

"Once you eliminate the impossible, whatever remains, no matter how improbable, must be the truth."
|
«
Return to General Discussions
|
1 view|%1 views
| Free forum by Nabble | Edit this page |

