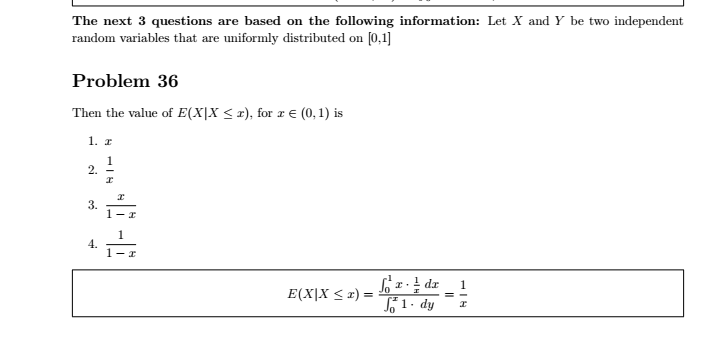
How is this possible? Could anyone solve this for me? I got x/2 as the answer. Moreover, isn't it possible to eliminate other options based on the fact that the expectations wouldn't be greater than 1 since x lies in the interval 0 and 1- which conveniently makes the last three options invalid. Where have I gone wrong? Thanks.