expected value ques
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
expected value ques
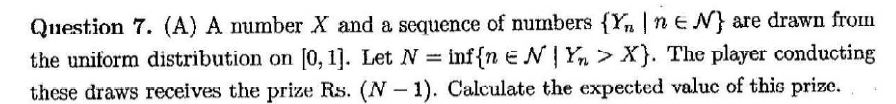 is the ans -1/2? I am not comfortable with my solution.
---
"You don't have to believe in God, but you should believe in The Book." -Paul Erdős |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: expected value ques
 I am getting E[P] = 100+n (where n is the total number of fish in the pond before 100 tagged fish are put in) will it be unbiased? or am I missing something and E[P] should come out to be n (the true population of the fish discounting the tagged fishes)??
---
"You don't have to believe in God, but you should believe in The Book." -Paul Erdős |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: expected value ques
|
Administrator
|
In reply to this post by Sinistral
E(N-1) cannot be -1/2 since N-1 always takes non-negative values.
Note that E(N -1) = E(N) - 1 (by linearity of expectation) Now let us find E(N) using E(N) = E(E(N|X)) E(N|X = x) = E(inf{n | Y(n) > x}|X = x) = E(inf{n | Y(n) > x}) (Since X and Y(n) are independent draws) Note that for 0 < x < 1, inf{n | Y(n) > x} has an interpretation: the number of trials until first success, where success is defined as Y(n) > x. And the probability of success in each independent trial is (1-x). E(N|X = x) = E(inf{n | Y(n) > x}) = 1(1-x) + 2x(1-x) + 3x^2(1-x) +...... = 1/(1-x) Now coming back to E(N): E(N) = E(E(N|X)) = E(1/(1-X)) = ∞ (infinity) Thus, E(N-1) = ∞. |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: expected value ques
|
Administrator
|
In reply to this post by Sinistral
Let M be the number of fishes in the pond before 100 tagged fishes were released. Probability of catching a tagged fish in each trial will then become 100/(100+M). Number of fishes (N) you catch till you get the tagged one is a geometric random variable with probability of success in each trial equal to 100/(100+M). Thus,
E(N) = (100+M)/100. M is estimated using P = 100 x N. For P to be an unbiased estimator of M, it must be the case that E(P) = M. Clearly, E(P) = 100(E(N)) = 100 + M ≠ M Thus, the estimator P is biased. V(P) = 10000(V(N)) = M(100+M) |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: expected value ques
|
This post was updated on Jun 08, 2013; 5:02am.
In reply to this post by Amit Goyal
why is the probability of success in each independent trial (1-x)? and yes thank u so much :)
---
"You don't have to believe in God, but you should believe in The Book." -Paul Erdős |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: expected value ques
|
oh got it!! :)
thanx again :)
---
"You don't have to believe in God, but you should believe in The Book." -Paul Erdős |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: expected value ques
|
In reply to this post by Amit Goyal
I didn't get how the probability of catching a tagged fish in each trial is the same. I thought P(N=2) would be (M/100+M)*(100/99+M). Are we approximating it by an infinite population?
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: expected value ques
|
In reply to this post by Sinistral
Also, Sinistral, would you mind sharing with us where you got this question from?
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: expected value ques
|
Administrator
|
In reply to this post by anon_econ
It is given in the question: You keep catching and releasing the fish until you get the tagged one.
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: expected value ques
|
Oops. I missed that. Thanks.
|
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: expected value ques
|
Hey Sinstral,
These questions are from DSE option-B paper..Isn't it????
M.A Economics
Delhi School of Economics 2013-15 Email Id:sumit.sharmagi@gmail.com |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: expected value ques
|
In reply to this post by anon_econ
2004 Option B Ques 8(B)
---
"You don't have to believe in God, but you should believe in The Book." -Paul Erdős |
Reply |
Threaded

Open this post in threaded view
|
More ♦
♦
| Loading... |
| Reply to author |
| Edit post |
| Move post |
| Delete this post |
| Delete this post and replies |
| Change post date |
| Print post |
| Permalink |
| Raw mail |
       |
Re: expected value ques
|
Thanks!
|
«
Return to General Discussions
|
1 view|%1 views
| Free forum by Nabble | Edit this page |

