DSE 2015 answer

[varnika1880]

Guys plz help with 17/19

[knowpraveen]

Static expectation means the expectation of a variable in one period is going to be equal to the actual value of the variable in the previous period, which brings us to the relation Ye(t+1)=Yt.

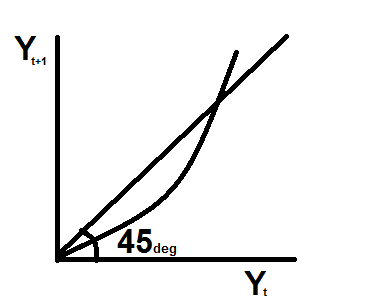
We have Y(t+1)=C(t+1)+I(t+1) which means Y(t+1)=aYe(t+1)+yYe(t+1)^2 where Ye is the expectation, a is alpha and y is gamma.

Let's see what kind of a function Y(t+1) is. It's a convex function which passes through a line through origin when Y=1-a/y.

Being a convex function, in the long run, Y(t+1)=Y(t) along the line through the origin, Y(t+1)<Yt for the region below Y<1-a/y and it's the opposite in the other case, which  means the value of Y in the long run approaches 0 or infinity depending upon whether Y< or > 1-a/y.

Draw a graph for clarity.

[knowpraveen]

In the case of rational expectations, the long run value of Y(t+1) and Yt are the same. It's also the same in the case of adaptive expectations, just in case.

This plainly implies Yt=aYt+yYt^2. Therefore, the long run equilibrium value of Y is 1-a/y

[varnika1880]

Thnx a lot .....

[knowpraveen]

Anytime. Could you please help me with the problem I just posted?

[varnika1880]

I already did ...can u plz attach a graph to this ...it's confusing this way ...thank

[knowpraveen]

[varnika1880]

Thnx

[Rautparul]

Hi, Where to study Vector from? Like that asked in question 9!!

[Abhitesh]

You can refer in Simon & blume,  I.n herstien. They don't deal with affine independence specifically but sufficient for isi-dse.

On 04-Jan-2017 3:53 PM, "Rautparul [via Discussion forum]" <[hidden email]> wrote:

Hi, Where to study Vector from? Like that asked in question 9!!

---

If you reply to this email, your message will be added to the discussion below:

http://discussion-forum.2150183.n2.nabble.com/DSE-2015-answer-tp7600806p7603020.html

To unsubscribe from DSE 2015 answer, click here.
NAML

[Rautparul]

Can somebody help with question 12?

[Rautparul]

Question 16--
IMG_20170108_153950.jpgI solved and got answer as option d but they saying option b.. whats wrong?

[Abhitesh]

Your answer is correct.
Check each options
A. (n+1)p=n(1+p) => np+p=n+np => p=n ; not possible.
B. np=(n-1)(1+p) = n-1+np-p => p=n-1 ; not possible.
C. np cannot be a position integer if (n+1)p is a positive integer.

[Rautparul]

Yes, and that's why I am not able to get why college has posted option b as Answer..

Can you help with question 43? 

 

I am stuck on how to solve SIgma (300 - 50Di - 262.5 )^2. 

On Mon, Jan 9, 2017 at 4:00 PM, Abhitesh [via Discussion forum] <[hidden email]> wrote:

Your answer is correct.
Check each options
A. (n+1)p=n(1+p) => np+p=n+np => p=n ; not possible.
B. np=(n-1)(1+p) = n-1+np-p => p=n-1 ; not possible.
C. np cannot be a position integer if (n+1)p is a positive integer.

---

If you reply to this email, your message will be added to the discussion below:

http://discussion-forum.2150183.n2.nabble.com/DSE-2015-answer-tp7600806p7603032.html

To unsubscribe from DSE 2015 answer, click here.
NAML

[Abhitesh]

Take Di=1 for i=1 to 150 and Di=0 for i=151 to 200
So we get
150*(12.5)^2 + 50*(37.5)^2

How did you solve question 42?

[Rautparul]

Thanks for that! :)

I am not sure about 42. I tried searching but didn't get anything satisfying enough. 

On Tue, Jan 10, 2017 at 5:42 PM, Abhitesh [via Discussion forum] <[hidden email]> wrote:

Take Di=1 for i=1 to 150 and Di=0 for i=151 to 200
So we get
150*(12.5)^2 + 50*(37.5)^2

How did you solve question 42?

---

If you reply to this email, your message will be added to the discussion below:

http://discussion-forum.2150183.n2.nabble.com/DSE-2015-answer-tp7600806p7603037.html

To unsubscribe from DSE 2015 answer, click here.
NAML

[Rautparul]

Can anyone write the equation for Question 56???

What is the condition for steady state long run??

It will be really great if u can post solution from 54 to 59

[amadv]

Thanks